Depth to water table correction for initial carbon-14 activities in groundwater mean residence time estimation

Carbon-14 (¹⁴C) is routinely used to determine mean residence times (MRTs) of groundwater. ¹⁴C-based MRT calculations typically assume that the unsaturated zone is in equilibrium with the atmosphere, controlling the input ¹⁴C activity. However, multiple studies have shown that unsaturated zone ¹⁴C activities are lower than atmospheric values. Despite the availability of unsaturated zone ¹⁴C data, no attempt has been made to generalise initial ¹⁴C activities with depth to the water table. We utilise measurements of unsaturated zone ¹⁴C activities from 13 studies to produce a ¹⁴C–depth relationship to estimate initial ¹⁴C activities. The technique only requires the depth to the water table at the time of sampling or an estimate of depth to water in the recharge zone to determine the input ¹⁴C activity, making it straightforward to apply. Applying this new relationship to two Australian datasets (113 ¹⁴C measurements in groundwater) shows that MRT estimates were up to 9250 years younger when the ¹⁴C–depth correction was applied relative to conventional MRTs. These findings may have important implications for groundwater samples that suggest the mixing of young and old waters and the determination of the relative proportions of young and waters, whereby the estimated fraction of older water may be much younger than previously assumed. Owing to the simplicity of the application of the technique, this approach can be easily incorporated into existing correction schemes to assess the sensitivity of unsaturated zone ¹⁴C to MRTs derived from ¹⁴C data.

1 Introduction

Environmental tracers are widely used to estimate both groundwater residence times (e.g. Love et al., 1994; Plummer and Sprinkle, 2001; Cartwright and Morgenstern, 2012; Jurgens et al., 2012) and recharge rates (e.g. Leaney and Allison, 1986; Cartwright et al., 2007; Gillon et al., 2009; Wood et al., 2015) that are important to effectively manage groundwater resources. Groundwater tracers can be used to determine mean residence times (MRTs) from tens to hundreds of years (e.g. tritium or CFCs), thousands to tens of thousands of years (e.g. carbon-14) to millions of years (e.g. helium-4 or chlorine-36). In particular, carbon-14 (¹⁴C) is widely used as a groundwater tracer owing to the ubiquitous presence of dissolved inorganic carbon in groundwater. With a half-life of 5730 years, ¹⁴C can be used to determine residence times of the order of 1000 to 30 000 years, which encompasses the range of residence times in many regional aquifers (Clark and Fritz, 1997). The use of accelerator mass spectrometry since the 1990s has significantly reduced the volumes of water required and has allowed highly precise measurements of ¹⁴C activities (of the order of ±1 %), further facilitating the use of ¹⁴C as a groundwater tracer (Cartwright et al., 2020).

Although atmospheric ¹⁴C activities have varied over time (e.g. Clark and Fritz, 1997; Cartwright et al., 2017), most studies of regional groundwater assume that prior to the atmospheric bomb testing in the 1960s they were constant at 100 % modern carbon (pmC). This approach yields so-called conventional radiocarbon ages in years before present (BP) where AD 1950 = 0 years BP (Clark and Fritz, 1997; Plummer and Glynn, 2013; Cartwright et al., 2020). The subsequent input of ¹⁴C-free C from calcite dissolution within aquifers may lower ¹⁴C activities, and several schemes based on statistical corrections, major ion geochemistry, and stable and radioactive isotopes exist to correct for this (Ingerson and Pearson, 1964; Vogel, 1967; Tamers, 1967; Mook, 1972; Fontes and Garnier, 1979; Clark and Fritz, 1997; Coetsiers and Walraevens, 2009; Han and Plummer, 2016; McCallum et al., 2018). Locally geogenic CO₂ and/or the oxidation of old organic matter may also lower ¹⁴C activities (e.g. Clark and Fritz, 1997; Cartwright et al., 2017, 2020).

Further complications in the use of ¹⁴C to estimate MRTs relate to the input function of ¹⁴C into the subsurface. ¹⁴C activities of CO₂ in the unsaturated zone are typically assumed to be in equilibrium with the atmosphere at the time of recharge (e.g. Mazor, 2004). However, ¹⁴C activities of CO₂ in the unsaturated zone (¹⁴C_uz) are commonly far lower than those of the atmosphere (e.g. Reardon et al., 1979; Haas et al., 1983; Thorstenson et al., 1983; Bacon and Keller, 1998; Carmi et al., 2009; Wood et al., 2014). For example, Carmi et al. (2009) note that ¹⁴C_uz activities were approximately 54 % of the atmospheric values in a coastal aquifer in Israel. Similar low ¹⁴C_uz activities that decrease with depth below the land surface have been recorded elsewhere (e.g. Yang et al., 1985; Walvoord et al., 2005; Wood et al., 2014). Despite the important role of the unsaturated zone in controlling input ¹⁴C activities, this issue is rarely discussed, and no attempt has been made to produce a generalised relationship that relates initial ¹⁴C activities to depth to the water table.

The aim of this study is to produce a general relationship between ¹⁴C_uz activity and the depth below surface to facilitate the estimation of input ¹⁴C activities to estimate MRTs. We produce a relationship using ¹⁴C_uz and sample depth data from 13 studies across North America, Europe, the Middle East, and Australia. We provide a demonstration of the newly presented relationship using datasets from the Limestone Coast and Ovens/Goulburn–Broken catchments in Australia to estimate MRTs. For the Ovens/Goulburn–Broken catchments dataset, we also present tritium data to provide supporting information for ¹⁴C-based calculations of MRTs. This work provides a simple-to-use approach to determine input ¹⁴C activities for groundwater MRT calculations that requires no additional measurement of groundwater chemistry. This approach can be incorporated into existing correction schemes to assess sensitivity of ¹⁴C_uz to MRTs derived from ¹⁴C data.

2 Methods

2.1 Unsaturated zone data collation

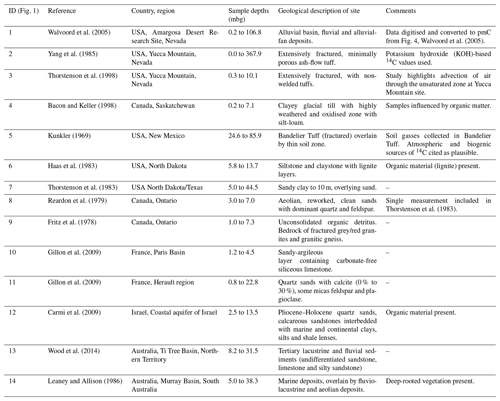
¹⁴C_uz activities were collated from the following sources: Kunkler (1969); Fritz et al. (1978); Reardon et al. (1979); Haas et al. (1983); Gillon et al. (2009); Thorstenson et al. (1983); Yang et al. (1985); Leaney and Allison (1986); Bacon and Keller (1998); Thorstenson et al. (1998); Walvoord et al. (2005); Carmi et al. (2009); Wood et al. (2014). A total of 181 ¹⁴C_uz activities were collated from 14 sites across North America, Europe, the Middle East, and Australia (Fig. 1a). ¹⁴C_uz activities were typically presented in tabular format in the original studies. In cases where data were presented graphically, ¹⁴C_uz activities were obtained by digitising plotted results.

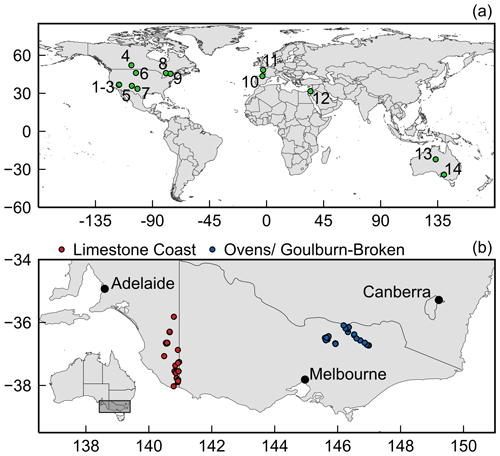
Figure 1 Locations of ¹⁴C data used. (a) Locations of studies of unsaturated zone ¹⁴C (¹⁴C_uz), where 1 is Walvoord et al. (2005), 2 is Yang et al. (1985), 3 is Thorstenson et al. (1998), 4 is Bacon and Keller (1998), 5 is Kunkler (1969), 6 is Haas et al. (1983), 7 is Thorstenson et al. (1983), 8 is Reardon et al. (1979), 9 is Fritz et al. (1978), 10 and 11 are Gillon et al. (2009), 12 is Carmi et al. (2009), 13 is Wood et al. (2014), and 14 is Leaney and Allison (1986). (b) Locations of groundwater ¹⁴C (¹⁴C_gw) data. Red markers show locations of ¹⁴C_gw samples from the Limestone Coast region of South Australia. Blue markers show locations of ¹⁴C_gw samples from the Ovens/Goulburn–Broken catchments, Victoria.

The ¹⁴C_uz study sites are predominantly located in North America (9 out of the 14 sites considered here), with 2 study sites from France (Gillon et al., 2009), 2 sites from Australia (Leaney and Allison, 1986; Wood et al., 2014) and 1 site in Israel (Carmi et al., 2009). A summary of the collated datasets is provided in Table 1. The complete ¹⁴C_uz dataset is available in Table S1 in the Supplement.

Table 1 Summary of studies of ¹⁴C_uz. Sample depths are presented in metres below ground (mbg).

2.2 Saturated zone data collation

¹⁴C activities of groundwater samples (¹⁴C_gw) were collated from the Limestone Coast region in South Australia and the Ovens/Goulburn–Broken catchments in Victoria (Fig. 1b). Samples of ¹⁴C_gw for the Limestone Coast region were collated from Love et al. (1994), Dogramaci (1998), Brown et al. (2001), van den Akker (2006), Wood (2011), SKM (2012), Turnadge et al. (2013) and SA Water (2020). ¹⁴C_gw activities from the Ovens/Goulburn–Broken catchments are from Cartwright et al. (2007) and Cartwright and Morgenstern (2012). In total, 51 samples were collated from the Limestone Coast and 62 samples from the Ovens/Goulburn–Broken catchments (Fig. 1b). The Ovens/Goulburn–Broken catchments dataset also includes ³H activities, which are useful for understanding mixing between old and young groundwater (e.g. Jasechko et al., 2017).

Depth to water (DTW, presented in metres below ground level, mbg) values to accompany the measured ¹⁴C_gw activities were included in Cartwright et al. (2007) and Cartwright and Morgenstern (2012) for the Ovens/Goulburn–Broken catchments. For the Limestone Coast, DTW values were determined as follows: if detailed time series of DTW were available for the sampled well, the measurement recorded as close in time to the ¹⁴C_gw sampling was used. Where this was not possible, the average DTW for the sample year was used in the sample well or a nearby well. All ¹⁴C_gw and DTW data for the groundwater samples from the Limestone Coast and Ovens/Goulburn–Broken catchments are provided in Table S2 in the Supplement. It is likely that the DTW in the recharge zone is more relevant. One approach could have been to determine DTW from spatially mapped water levels (e.g. Wood et al., 2017). Nonetheless, the simple approach to estimate DTW from the sampled wells allows for a demonstration of the methods outlined here.

2.3 Data analysis

The unsaturated zone sample depth–¹⁴C_uz relationship was produced by fitting the ¹⁴C_uz and sample depth data (Table S1) using the curve_fit function in the scipy.optimize library and the nominal_values function from the uncertainties.unumpy libraries in Python. The curve fitting approach was used to determine the coefficients a and b in the equation ¹⁴C_uz=a exp(bz). This approach also was used to find the best fit to the data as well as to produce upper and lower bounds on the best fit relationship based on the standard deviation of the observed data.

Not all collated ¹⁴C_uz data were used to produce the ¹⁴C–depth relationships, with three principal reasons for the exclusion of data: (1) ¹⁴C_uz activities were influenced by the presence of high levels of organic material in the unsaturated zone; (2) ¹⁴C_uz activities were influenced by deep-rooted vegetation or (3) where modern atmospheric gases may have influenced the measured ¹⁴C_uz activities. Modern air can pollute samples either through the drilling process or through the advection of air through the unsaturated zone (e.g. Thorstenson et al., 1998). A description of whether or not data values were used in the fitting process and explanations for the omission of data values are provided in Table S1.

The compiled ¹⁴C_uz dataset (Table S1) includes the depth below the surface of the sample, the measured ¹⁴C, and the sample year. It is expected that the ¹⁴C_uz activities will be elevated where dissolved inorganic carbon influenced by the bomb peak activities has entered the subsurface. The actual input function of ¹⁴C will differ from atmospheric inputs, as the ¹⁴C is first cycled through vegetation, with significant delays between the uptake of ¹⁴C in trees and entering the unsaturated zone. For example, Fritz et al. (1978) measured ¹⁴C_uz values of 141±10 pmC in the unsaturated zone in 1975, after peak ¹⁴C activities of the order of 180 pmC in the atmosphere in the mid-1960s (Fig. S1, Hua et al., 2013). Owing to the abovementioned complexities, the sample date was not taken into account in the fitting process. Additionally, while many of the unsaturated zone studies also included δ¹³C measurements, these data were not utilised here as δ¹³C–depth profiles have been shown to be almost vertical with depth (e.g. Walvoord et al., 2005; Wood et al., 2017), containing little additional information.

¹⁴C-based MRTs (years) were determined from the groundwater ¹⁴C measurements using the simple radioactive decay equation (Clark and Fritz, 1997):

$$\begin{array}{}\text{(1)}& \mathrm{MRT}=-\mathrm{8267}\ln \left({\displaystyle \frac{{}^{\mathrm{14}}{C}_{\mathrm{gw}}}{{}^{\mathrm{14}}{C}_{i}q}}\right),\end{array}$$

where ¹⁴C_gw is the measured ¹⁴C activity in groundwater (pmC), ¹⁴C_i is the initial ¹⁴C activity of the recharging water (pmC) and q is the proportion of dissolved inorganic carbon that originated from groundwater recharge. While this approach neglects the fact that (1) water follows variable flow paths, (2) undergoes dispersion within the aquifer and also (3) assumes a uniform atmospheric ¹⁴C activity, it serves to illustrate the effects of variable ¹⁴C_uz. It is also the approach that is used in the majority of ¹⁴C studies (e.g. Cartwright et al., 2020). Again, for simplicity, we initially do not account for the input of ¹⁴C-free C from the aquifer matrix (i.e. q=1); the impacts of the addition of ¹⁴C-free C are discussed in Sect. 3.3.

MRTs were calculated from the measured ¹⁴C_gw activities using Eq. (1), firstly assuming that ¹⁴C_i=100 pmC to produce conventional MRT estimates. Secondly, ¹⁴C_i values determined from the DTW at the time of sampling using the DTW–¹⁴C relationship derived in Sect. 3.1 were used to calculate the MRTs. MRTs were also estimated using the upper and lower bounds of the fitted relationship, producing upper and lower bounds on the MRT estimates based on the DTW corrections. The calculation of MRTs ignores the time taken for water to infiltrate through the unsaturated zone. The timescales of infiltration are expected to be of the order of a few weeks to a few years, which is short relative to the several thousand-year timeframes of ¹⁴C-based MRTs.

The lower limit of MRTs that can be estimated from ¹⁴C analyses is of the order of 1000–2000 years (Clark and Fritz, 1997; Cartwright et al., 2020). Here, MRTs of less than 1000 years are considered to be “young water”. For visualisation purposes, MRTs of < 500 years are presented as 500 years as precise ¹⁴C-based MRTs cannot be determined for these samples. We adopt the Jasechko et al. (2017) definition of “fossil water” as water with MRTs that exceed 12 000 years, which corresponds to the beginning of the Holocene.

3 Results

3.1 Development of the depth–¹⁴C relationship

The 181 unsaturated zone ¹⁴C activities are shown in Fig. 2. Shallow ¹⁴C_uz data (< 20 mbg), particularly the shallow values with very low ¹⁴C activities (< 25 pmC), were excluded from the data-fitting process as the original articles suggest that these samples were influenced by oxidation of “old” (low ¹⁴C activity) organic matter in the unsaturated zone sediments (Fig. 2). Unsaturated zone studies with data that were excluded from the data-fitting process due to the presence of organic matter included Bacon and Keller (1998), Haas et al. (1983), and Carmi et al. (2009). Other ¹⁴C activities were influenced by deep-rooted vegetation (e.g. Leaney and Allison, 1986) and were also excluded from the data that were used in the fitting process. Finally, data from the Yucca Mountain sites were omitted from the fitting process. Thorstenson et al. (1998) proposed that advection of modern air through the unsaturated zone has produced elevated ¹⁴C_uz activities at the site. Thus, the relationships shown in Fig. 2 are representative of sedimentary basins and should generally not be applied where high organic matter or deep-rooted vegetation is present or where advection of modern air occurs in the unsaturated zone.

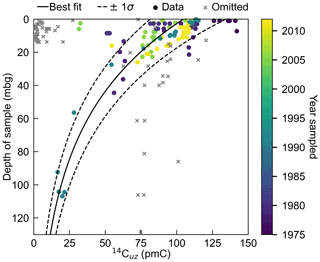
Figure 2 Relationship between pmC in the unsaturated zone (¹⁴C_uz) and depth below the surface for sedimentary basins. Data points omitted from the fitting process (grey crosses) were omitted due to the presence of high organic matter, deep-rooted vegetation, or where gasses were influenced by the advection of modern air into the unsaturated zone.

The best fit relationship (R²= 0.64) presented in Fig. 2 (solid line) can be represented mathematically as

$$\begin{array}{}\text{(2)}& {}^{\mathrm{14}}{C}_i=\mathrm{104.634}{e}^{-\mathrm{0.01693}z},\end{array}$$

where z is the depth below ground (mbg). The value 104.634 in Eq. (2) represents the ¹⁴C_i at z= 0, which is reasonably consistent with present atmospheric ¹⁴C activities (e.g. Hua et al., 2013). The upper and lower bounds in Fig. 2 (dashed lines) were based on ±1 standard deviation (σ). The upper and lower bounds' relationships can be represented mathematically as

$$\begin{array}{}\text{(3)}& {}^{\mathrm{14}}{\mathrm{C}}_i=\mathrm{133.422}{e}^{-\mathrm{0.01666}z}\end{array}$$

and

$$\begin{array}{}\text{(4)}& {}^{\mathrm{14}}{\mathrm{C}}_i=\mathrm{82.606}{e}^{-\mathrm{0.01720}z}.\end{array}$$

The selection of 1σ was used rather than the more commonly used 2σ (representing upper and lower 95 % confidence intervals) as the use of 2σ produced unrealistically high ¹⁴C_uz activities in the shallow zone (0–1 m). As with the application of other correction schemes, care should be taken in interpreting MRTs determined using Eqs. (2)–(4) to determine ¹⁴C_i values.

3.2 Application of the depth–¹⁴C relationship

Conventional MRTs determined assuming ¹⁴C_i= 100 pmC (Eq. 1) and those using the DTW correction (Eq. 2) for the Limestone Coast and Ovens/Goulburn–Broken catchments are presented in Fig. 3.

Figure 3 Calculated MRTs for the Limestone Coast (left column, red colours) and the Ovens/Goulburn–Broken catchments (right column, blue colours). Panels (a) and (b) show the difference in calculated MRTs for the uncorrected (Eq. 1, where ¹⁴C_i= 100 pmC, square markers) and depth-corrected ¹⁴C_i values (circle markers). Panels (a) and (b) use definitions of young water of ≤ 1000 years (e.g. Cartwright et al., 2020) and fossil water of ≥ 12 000 years (Jasechko et al., 2017). Panels (c) and (d) show the difference in MRTs between the use of 100 pmC and the value calculated in Eq. (2) against the measured ¹⁴C activity in groundwater (¹⁴C_gw). Marker colours in (c) and (d) are based on the depth to the water table (mbg) when the sample was collected.

The application of Eq. (2) to determine ¹⁴C_i values generally produced younger MRTs than the conventional MRTs (Fig. 3a, b). Exceptions to this observation occurred in cases where the DTW-corrected ¹⁴C_i values exceeded 100 pmC (which, following Eq. 2, occurs when the DTW < 2.67 mbg), which occurred more frequently in the Ovens/Goulburn–Broken catchments (Fig. 3b). The application of Eq. (2) to determine ¹⁴C_i led to 3 young water samples (out of 51) for the Limestone Coast, where the uncorrected residence times were > 1000 years (Fig. 3a). The greatest difference between the DTW-corrected and conventional MRTs for the Limestone Coast data was 9250 years, which corresponded to the ¹⁴C measurement with the deepest DTW (68.8 mbg, Fig. 3c). On average, the MRTs were approximately 1500 years younger for the DTW-corrected MRTs relative to the conventional MRTs for the Limestone Coast data (Fig. 3a, c).

The MRTs from the Ovens/Goulburn–Broken catchments (Fig. 3b, d) were generally much younger than the Limestone Coast samples. For the Ovens/Goulburn–Broken catchments, the greatest difference between the DTW-corrected and conventional MRTs was 5410 years, with an average difference of approximately 300 years. As was the case with the Limestone Coast samples, the largest difference in estimated residence times was produced for the sample with the largest depth to water at the time of sampling (41.3 mbg). The average difference between the DTW-corrected and conventional MRTs was skewed for the Ovens/Goulburn–Broken catchments by the fact that many of the calculated MRTs were young (< 500 years) for both the conventional and DTW-corrected approaches. Five samples for the Ovens/Goulburn–Broken catchments were deemed to be young (< 1000 years) after the application of the DTW correction. Interestingly for the Ovens/Goulburn–Broken catchments, one sample originally categorised as fossil water (> 12 000 years) was no longer classified as fossil with the application of the DTW-correction approach.

The MRTs presented in Fig. 3 used 100 pmC as the ¹⁴C_i value and best fit correction scheme to determine ¹⁴C_i (Eq. 2). Figure 4 includes the use of Eqs. (2)–(4) to determine ¹⁴C_i values, thus providing lower and upper bounds on the estimated MRTs from the DTW-correction approach. Tabulated values of the MRTs presented in Fig. 4 are available in Table S3.

Figure 4 Mean residence times determined using the best fit relationship (Eq. 2, circle markers), the upper limit (Eq. 3, upper whisker) and the lower limit (Eq. 4, lower whisker). For sample numbers (y axis); see Tables S2 and S3 in the Supplement. (a) MRTs from the Limestone Coast region and (b) MRTs from the Ovens/Goulburn–Broken catchments. In both panels, shading denotes the measured ¹⁴C_gw activities.

The application of Eqs. (3) and (4) to determine ¹⁴C_i increases the range in depth-corrected MRTs for both the Limestone Coast and Ovens/Goulburn–Broken catchments. For the Limestone Coast samples (Fig. 4a), two of the samples that were considered fossil water when ¹⁴C_i was calculated from the best fit equation (Eq. 2) were not considered fossil water if Eq. (4) (lower bound; see Fig. 2) was used. For the Limestone Coast data, $\mathrm{18}/\mathrm{51}$ samples were below the 500-year bounds placed on the minimum estimated MRT when Eq. (4) was used to determine ¹⁴C_i. The majority of MRT estimates for the Ovens/Goulburn–Broken catchments were young water when using Eq. (2) to determine ¹⁴C_i (Fig. 4b). Approximately 70 % of the samples from the Ovens/Goulburn–Broken catchments had estimated MRTs ranging between young water (500 years here) and ∼ 3000 years.

3.3 Comparisons between ¹⁴C-based residence time and tritium activities

The Ovens/Goulburn–Broken catchment ³H data (Table S2, Fig. 5) provide the opportunity to assess the ¹⁴C-based MRT estimates. Figure 5 shows the raw ¹⁴C and ³H activities. Combined ³H and ¹⁴C_gw data allow mixing to be identified (Le Gal La Salle et al., 2001; Favreau et al., 2002; Cartwright et al., 2007, 2017). The ³H peak produced by the atmospheric nuclear tests in the Southern Hemisphere was significantly lower than in the Northern Hemisphere, and ³H activities of groundwater recharged at that time are lower than those of modern rainfall (Morgenstern et al., 2010). Figure 5 shows ³H and ¹⁴C_gw activities in the Ovens and Goulburn–Broken groundwater (data from Table S2). Unsurprisingly, there is a general correlation between ¹⁴C_gw and ³H. The shaded fields (Fig. 5) show the predicted covariation of these isotopes for the case where no macroscopic mixing between old and young groundwater has occurred. This was constructed for a variety of flow geometries (i.e. using the exponential piston flow and dispersion models; see Cartwright et al., 2017), the ³H record of rainfall in Melbourne, and the Southern Hemisphere atmospheric ¹⁴C_gw record following Le Gal La Salle et al. (2001) and Cartwright et al. (2007, 2017). Samples lying outside these shaded regions most likely record the mixing between old and young groundwater. Closed system calcite dissolution lowers ¹⁴C activities (the lighter region in Fig. 5 is for 15 % calcite dissolution, i.e. q= 0.85). Samples lying to the right of the co-variance fields have relatively low ¹⁴C activities but measurable ³H and would generally be interpreted as mixtures of young recently recharged water and older water flowing through the aquifer. However, because an additional reduction of ¹⁴C_i may occur due to dilution of unsaturated zone ¹⁴CO₂, some of these samples may not show mixing. Samples to the left are likely over-corrected, whereby ¹⁴C_i values are too high (e.g. Cartwright et al., 2013).

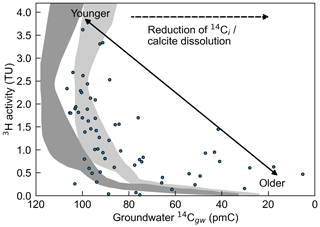
Figure 5 Comparisons between measured ³H and ¹⁴C_gw activities and MRTs for the Ovens/Goulburn–Broken catchments (see Fig. 2). Note that the x axis (¹⁴C_gw) has been inverted. Grey shading denotes the predicted covariation of ³H and ¹⁴C for the case where no macroscopic mixing between old and young groundwater has occurred, dark grey for q= 1 and light grey for q= 0.85. Dark grey shaded region from Cartwright et al. (2017).

4 Discussion

The DTW corrections (Eqs. 2–4) to estimate ¹⁴C_i values proposed here are strongly influenced at depth (> 60 m) by the omission of the ¹⁴C_uz data from the Yucca Mountain site (e.g. Kunkler, 1969; Yang et al., 1985). The Yucca Mountain data were excluded from the data-fitting process owing to complications induced by drilling at the site. Thorstenson et al. (1998) highlight that drilling in the 1980s provided conduits for modern atmospheric gases to enter the system, thus providing explanations for the elevated ¹⁴C_uz values at depths up to 400 m. The DTW corrections to estimate ¹⁴C_i presented here could be significantly revised, particularly at depths exceeding 60 m, through the inclusion of further ¹⁴C_uz data. The inclusion of the ¹⁴C_uz data from Kunkler (1969) and Yang et al. (1985) would lead to higher ¹⁴C_i values determined from Eq. (2) for depths exceeding 60 m (Eq. 2) and wider uncertainty bands (Eqs. 3, 4) over the same depth range. The exclusion of the (generally deep) Yucca Mountain data in the generation of the DTW-correction relationships had only a minor influence on the interpretations of MRTs in the Limestone Coast and Ovens/Goulburn–Broken catchments (Figs. 3, 4), owing to the depths to the water table at the time of sampling. This observation would hold even in the case where DTW values from the recharge zone rather than sampling wells were used. Of the 113 ¹⁴C_gw samples used to estimate MRTs, only 2 samples had water tables that were 30 m or more below the land surface. The fact that Eqs. (2)–(4) rely on data from a single study for depths below ∼60 mbg (e.g. Walvoord et al., 2005) is a limitation for the application of the DTW-correction approach outlined here for sites with deep water tables.

The DTW-correction approach presented here is straightforward to apply as it requires only a measurement of the depth to the water table at the time of sampling and does not require additional data. The application of the DTW correction was illustrated here using conventional ¹⁴C ages, but it could be easily incorporated into the input function for lumped parameter models or numerical models. For example, Wood et al. (2017) used the relationship between DTW and ¹⁴C_uz for the Ti Tree Basin (central Australia) to generate spatially variable ¹⁴C inputs in a regional-scale solute transport model.

The example applications presented here used the DTW at the sampling well used to estimate the ¹⁴C_i values. These DTW values are likely greater than the DTW at the recharge zone, at the time of recharge, leading to minor over-corrections of ¹⁴C_i values from Eqs. (2) to (4). For example, for Well ID 7022-128 (Sample ID = 16, ¹⁴C_gw= 13.35 pmC, DTW = 27.47 m; see Table S3), the MRT using Eq. (2) was 13 180 years. If the DTW was assumed to be 5 m shallower (22.47 m), the MRT increased to 13 880 years (700 years, or ∼5 %). Given that Eqs. (2)–(4) are straightforward to implement, the impact of uncertainty on the DTW could be easily investigated. However, ignoring the role of the unsaturated zone completely can lead to significant over-estimation of MRTs (see Fig. 3). For example, the relationship presented in Fig. 6 shows the potential errors in MRT estimates from the assumption that ¹⁴C_i= 100 pmC, where ¹⁴C_i equals the value on the x axis. To relate the error in MRT estimates to the depth to the water table, the corresponding depths to the water table from Eq. (2) are presented on the secondary x axis. Figure 6 demonstrates that the assumption of ¹⁴C_i= 100 pmC for a 24 m-deep water table could lead to an over-estimation of MRTs of the order of 3000 years. Errors in estimated MRT can have major implications for recharge estimation. For example. Wood et al. (2015) highlighted that errors in MRTs caused by assumptions of initial ¹⁴C activities can lead to errors in recharge estimates that exceed an order of magnitude. Furthermore, ignoring the possibilities of low ¹⁴C_uz values complicates the assessment of mixing using joint ¹⁴C and ³H data.

Figure 6 Maximum difference in calculated MRT (y), where ¹⁴C_iq on the x axis is used, relative to the case where it is assumed to be 100 pmC. The secondary x axis shows indicative water depths that correspond to ¹⁴C_iq values shown on the lower x axis according to Eq. (2). The result assumes q= 1.

5 Concluding remarks

Due to advances in technology, particularly the development of accelerator mass spectrometry in the case of ¹⁴C, hydrogeologists can measure radioisotopes with higher throughput, lower detection limits, and higher precision than was the case 20 to 30 years ago. This has permitted more comprehensive studies to be carried out and led to a rapidly growing database of ¹⁴C measurements. The continuing realisation, however, that there are numerous processes aside from radioactive decay that affect ¹⁴C activities means that we are less certain in translating these data into useful parameters such as residence times. That some of these processes, such as those discussed here, occur in the unsaturated zone, prior to recharge, adds to the complexity as these are difficult to resolve using groundwater data alone. Previous studies have focused on the importance of calculating q in mean residence time estimates (e.g. Coetsiers nd Walraevens, 2009; Han and Plummer, 2016). Here, the importance of accounting for processes in the unsaturated zone is demonstrated.

The analysis of ¹⁴C–depth relationships in the unsaturated zone is currently hampered by a relatively small number of studies, especially at the depths exceeding 60 m. However, given that the impact on calculated mean residence times may exceed that caused by closed system calcite dissolution, further attention is warranted. Data from a wider range of environments would also help in assessing the variability of these relationships.