Impact of detention dams on the probability distribution of floods

Detention dams are one of the most effective practices for flood mitigation. Therefore, the impact of these structures on the basin hydrological response is critical for flood management and the design of flood control structures. With the aim of providing a mathematical framework to interpret the effect of flow control systems on river basin dynamics, the functional relationship between inflows and outflows is investigated and derived in a closed form. This allowed the definition of a theoretically derived probability distribution of the peak outflows from in-line detention basins. The model has been derived assuming a rectangular hydrograph shape with a fixed duration and a random flood peak. In the present study, the undisturbed flood peaks are assumed to be Gumbel distributed, but the proposed mathematical formulation can be extended to any other flood-peak probability distribution. A sensitivity analysis of parameters highlighted the influence of detention basin capacity and rainfall event duration on flood mitigation on the probability distribution of the peak outflows. The mathematical framework has been tested using for comparison a Monte Carlo simulation where most of the simplified assumptions used to describe the dam behaviours are removed. This allowed demonstrating that the proposed formulation is reliable for small river basins characterized by an impulsive response. The new approach for the quantification of flood peaks in river basins characterized by the presence of artificial detention basins can be used to improve existing flood mitigation practices and support the design of flood control systems and flood risk analyses.

1 Introduction

During the last decades, the growing number of hydrological extremes have raised economic losses and risk perception at the global scale (Peduzzi, 2005; Di Baldassarre et al., 2010; Winsemius et al., 2016). The impact of natural disasters has been quantified in a recent study by Wallemacq and Below (2015), which is based on the Emergency Events Database (EM-DAT). According to EM-DAT, flooding impacted on nearly 2.5 billion people in the period 1994–2013. A more recent study by Munich Re (NatCatSERVICE, Munich Reinsurance Company (MunichRe), 2020) reported 3798 flash flood events that produced economic losses of about USD 592 billion and killed around 100 000 people worldwide during the last two decades (2000–2018).

In this context, climate change and anthropic activities are probably accelerating the number of extremes (Fischer and Knutti, 2016​​​​​​​; Papalexiou and Montanari, 2019). In fact, these two factors are significantly modifying river basin hydrology (Di Baldassarre et al., 2017), which now requires renewed methods and techniques. Therefore, the need to find appropriate measures for flood hazard prevention and mitigation is becoming more and more pressing.

Flood risk management is a complex challenge for hydrologists that need to identify dynamic solutions for flood mitigation. Plate (2002) underlined the need to update flood mitigation plans according to the changing conditions (e.g. climate, populations, land use). Hence, the design of flood mitigation systems requires rapid methodologies to evaluate scenarios and intervention measures and obtain effective flood risk management strategies (Franzi et al., 2016).

One of the most effective actions for the attenuation of peak discharges is represented by a detention basin system or coordinated reservoir operations (e.g. UDFCD, 2016; Jacob et al., 2019; Seibert et al., 2014). The proper design of a detention basin is an extremely complex task given the large number of factors that are involved in the decision process: technical engineering features of the structure, site selection and environmental characteristics. For this reason, Bellu et al. (2016) proposed an innovative method to optimize the dimensioning and site selection of a flood mitigation system. The method follows three steps that include a preliminary sizing, a site location and optimization according to environmental objectives. Nevertheless, the sizing of the detention volume is based, in most of the cases, on a given design flood event neglecting the random nature of the phenomena and the impact of the structure on a large spectrum of events.

The flood attenuation caused by the presence of artificial reservoirs is influenced by hydrologic and hydraulic factors, such as flood wave shape and duration, as well as by the storage capacity and geometric parameters of the detention basin. Therefore, it is crucial to build a mathematical scheme able to interpret the functional relationships, even in a simplified form, among the mentioned variables.

With this aim, the present article introduces a theoretically derived probability distribution (TDD) of detention basin outflows, which is obtained assuming the incoming flood peaks randomly distributed and characterized by rectangular hydrographs of fixed duration (see e.g. Manfreda et al., 2018). In the present case, flood distribution is assumed to be Gumbel for the sake of simplicity, but the proposed approach can be applied to any flood-peak probability distribution. Performances of the proposed method are investigated under different configurations and are tested with a numerical simulation of flood mitigation. This method can be applied to improve existing flood mitigation approaches and support the design of flood control systems, flood risk and damage analyses.

This paper is organized as follows: in Sect. 2, the conceptual scheme of the detention basin adopted to derive the mathematical formulation of outflows is described. Furthermore, the hydraulic concepts and assumptions to analytically compute the derived probability distribution of the peak outflows are introduced; in Sect. 3, the proposed methodology is tested under different reservoir configurations and compared with the results of the numerical simulations, and a brief description of the numerical model is also provided; in Sect. 4 main findings and results are discussed.

2 The conceptual scheme

In-line detention dams (also known as flow-through dams) are constructed solely with the purpose of flood control and mitigation of flood risks in downstream communities and ecosystems. Unlike reservoir dams, which are primarily built for water storage or power generation, the spillway (opening) is located at the same height as the riverbed level, allowing the river to continue its natural flow under normal conditions. When water levels rise above the spillway, the dam restricts the amount flowing through the opening, decreasing peak flow. Since detention dams minimally affect rivers' natural flows, under normal conditions negative environmental and socioeconomic impacts, such as sediment accumulation, restriction of water flow to downstream communities and ecosystems, and breaching during very extreme flood events, can be minimized or avoided altogether.

The schematization of the detention dam has been simplified with the aim to obtain a mathematical description of the outflows associated with a given hydrograph. In particular, we assumed that the dam body has two openings: a low-level opening at the basement and a crest spillway. The former is assumed to let pass the flow below a given control value, while the latter starts functioning only when the volume of the dam is completely filled up to the crest level. Thereafter, the water starts to flow from both the openings, and the control is mainly exerted by the basin volume above the emergency spillway (crest level). This scheme can be described in closed form that may help the construction of a derived probability distribution of the outflow from a detention dam.

2.1 The hydraulic characteristics of the problem

Let us first introduce the key equations controlling the dynamics of a system like the one under study. The first equation to introduce is represented by the stage-storage capacity curve which is able to describe the morphology of the gorge closed by a specific dam. The function is generally represented by a power law:

$$\begin{array}{}\text{(1)}& W\left(h\right)=w_{\mathrm{1}}{h}^n,\end{array}$$

where W(h) [m³] is the water storage of detention basin, w₁ [m³⁻ⁿ] is the parameter of the stage-storage capacity curve, h [m] is the water level in the reservoir and n [–] is the exponent influenced by the shape of the control volume. The exponent ranges between 1 and 4.5, where 1 is associated with a prismatic geometry with vertical surrounding walls and 4.5 is associated with a more complex morphology closed by more gentle lateral slopes.

The streamflow of the river system reaching the reservoir is altered by it based on the hydraulic characteristics of the dam. The outflow will be controlled by the amount of water accumulated in the system according to the continuity equation:

$$\begin{array}{}\text{(2)}& {\displaystyle \frac{\mathrm{d}W\left(t\right)}{\mathrm{d}t}}=Q_{\mathrm{in}}\left(t\right)-Q_{\mathrm{out}}\left(t\right),\end{array}$$

where Q_in(t) is the incoming flux and Q_out(t) is the outflow from the reservoir.

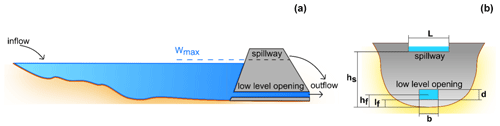
Figure 1 Sketch of a detention basin section with a basin capacity W_max, composed of a low-level opening, with an area equal to the product between b and d, and a crest spillway of length L and height h_s.

The outflow can be computed using the traditional formulation of hydraulics based on the variation of the water level, h, in the reservoir, which can be derived from the stage-storage capacity curve (Eq. 1) and continuity equation (Eq. 2). In particular, we can assume that the outflow for a simple scheme with two openings (the low-level opening and the emergency spillway) and the characteristics described in Fig. 1 may vary according to four different discharge laws expressed as a function of the reservoir water level stage. The outflow can be described as follows:

$$\begin{array}{}\text{(3)}& Q_{\mathrm{out}}=\left\{\begin{array}{l}\mathrm{0},\mathrm{0}<h\le l_{\mathrm{f}}\\ {\mathit{\mu }}_{\mathrm{s}}b\sqrt{\mathrm{2}g}{\left(h-l_{\mathrm{f}}\right)}^{\frac{\mathrm{3}}{\mathrm{2}}},l_{\mathrm{f}}<h\le h_{\mathrm{f}}\\ {\mathit{\mu }}_{\mathrm{f}}A\sqrt{\mathrm{2}g}{\left(h-h_{\mathrm{f}}\right)}^{\frac{\mathrm{1}}{\mathrm{2}}},h_{\mathrm{f}}<h\le h_{\mathrm{s}}\\ {\mathit{\mu }}_{\mathrm{f}}A\sqrt{\mathrm{2}g}{\left(h-h_{\mathrm{f}}\right)}^{\frac{\mathrm{1}}{\mathrm{2}}}+{\mathit{\mu }}_{\mathrm{s}}L\sqrt{\mathrm{2}g}{\left(h-h_{\mathrm{s}}\right)}^{\frac{\mathrm{3}}{\mathrm{2}}},h>h_{\mathrm{s}},\end{array}\right.\end{array}$$

where μ_f [–] is the coefficient of discharge of the low-level opening at the basement (suggested values may range between 0.6 (assuming a thin sharp edge) and 0.8 (assuming a wall with thickness 2 times larger than the opening height)), A [m²] is the area of the low-level opening (i.e. the product of the opening width, b [m], and the minimum dimension between the top of the flow surface at the opening exit and the bottom of the opening), μ_s [–] is the coefficient of discharge of the spillway crest (suggested values range around 0.3–0.4 based on the geometry of the weir), l_f [L] is the height of the low-level opening at the basement, L [m] is the effected crest length, h_f [m] is the height of the barycentre of the low-level opening, h_s [m] is the height of the spillway crest, and g [m/s²​​​​​​​] is the acceleration due to gravity.

The above expression includes the three main configurations that may occur in the proposed scheme with the increase of the water level stage in the reservoir. A detailed description of the scheme proposed along with graphical indication of the parameters meaning is given in Fig. 1. In particular, the opening at the bottom does not exert a significant control on the incoming flow as long as the water level does not generate a submergence of the opening (i.e. as long as h≤h_s). The water flow starts to be limited when the water stage reaches the value h_f, after which the opening is submerged. This allows filling the storage volume of the reservoir up to the level of the crest spillway. After this stage, the reservoir tends to operate a mitigation that is influenced by the water storage capacity of the reservoir above the crest level and the hydraulic characteristics of the spillway. An example of flood mitigation obtained via numerical simulation is given in Fig. 2, which provides a comparison between a synthetic hydrograph and the outflow from a detention dam.

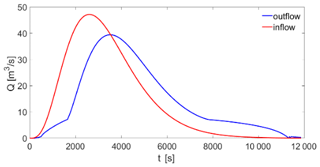
Figure 2 Example of flood mitigation induced by the presence of a detention basin obtained via numerical simulation. Other parameters are b=1 m; d=1 m; n=1.9; $h_{\mathrm{f}}=l_{\mathrm{f}}+d/\mathrm{2}$; h_s=4; μ_f=0.85; μ_s=0.385; L=3 m, W_max=15 000 m³.

For the scope of the present study, the outflow has been simplified through the following set of equations:

$$\begin{array}{}\text{(4)}& Q_{\mathrm{out}}=\left\{\begin{array}{l}{Q}_{\mathrm{in}},\mathrm{0}<h\le h_{\mathrm{f}}\\ Q_{\mathrm{c}},h_{\mathrm{f}}<h\le h_{\mathrm{s}}\\ {\mathit{\mu }}_{\mathrm{f}}A\sqrt{\mathrm{2}g}{\left(h-h_{\mathrm{f}}\right)}^{\frac{\mathrm{1}}{\mathrm{2}}}+{\mathit{\mu }}_{\mathrm{s}}L\sqrt{\mathrm{2}g}{\left(h-h_{\mathrm{s}}\right)}^{\frac{\mathrm{3}}{\mathrm{2}}},h>h_{\mathrm{s}},\end{array}\right.\end{array}$$

where Q_c is the control value of discharge that is computed using the discharge equation of the submerged opening and assuming h=h_s.

Assuming a rectangular hydrograph of the incoming flow, it is possible to derive the peak flow associated with an incoming flood peak. Following the simplifying assumption given in Eq. (4), the outflow is not affected by the presence of the dam for lower streamflow values, while it is modified when the inflow exceeds the control discharge. In particular, the outflow remains almost constant as long as the reservoir is filled and, thereafter, it is controlled by the crest spillway. In this last configuration, it is possible to use the linear reservoir concept for the water volume accumulated above the elevation of the crest spillway.

In order to estimate the peak flow associated with a specific rectangular hydrograph of constant discharge equal to Q_max, we should recall the expression of the peak flow generated by a simple linear reservoir, which can be described as follows:

$$\begin{array}{}\text{(5)}& Q_{\mathrm{lam}}=Q_{\max }(\mathrm{1}-e^{-t_{\mathrm{p}}/k}),\end{array}$$

where t_p [s] is the event duration, and k [s] is the delay constant of the conceptual linear reservoir.

The above equation should be modified, in the present case, considering that the flood event should fill the dam water storage capacity (or detention basin) before reaching the crest spillway. Therefore, the crest spillway will be activated only after a time:

$$t_{\mathrm{filling}}={\displaystyle \frac{W_{\max }}{(Q_{\max }-Q_{\mathrm{c}})}},$$

where W_max is the volume of water accumulated in the dam at the crest level h_s.

When the volume below the spillway crest is totally filled, the crest spillway starts functioning for discharge values above the control discharge that is released at the bottom. Therefore, the peak outflow, Q_p,out, assumes the following form:

$$\begin{array}{}\text{(6)}& \begin{array}{rl}{Q}_{\mathrm{p},\mathrm{out}}& =Q_{\mathrm{c}}+(Q_{\max }-Q_{\mathrm{c}})\\ & \left(\mathrm{1}-\mathrm{Exp}\left[-\left(t_{\mathrm{p}}-{\displaystyle \frac{W_{\max }}{\left(Q_{\max }-Q_{\mathrm{c}}\right)}}\right)/k_{\mathrm{eq}}\right]\right),\end{array}\end{array}$$

where k_eq [s] is the equivalent delay constant of the conceptual reservoir associated with the outflow. This parameter can be derived exploiting the characteristics of the spillway and the stage-storage capacity curve. In particular, according to the linearity concept, the two functions should have the same exponent. Under such a hypothesis, the parameter k_eq can be estimated as

$$\begin{array}{}\text{(7)}& k_{\mathrm{eq}}={\displaystyle \frac{w_{\mathrm{2}}}{{\mathit{\mu }}_{\mathrm{s}}L\sqrt{\mathrm{2}g}}},\end{array}$$

where w₂ is the coefficient of the rescaled stage-storage capacity curve above the crest level. Such a parameter should be computed in order to get the best approximation of the function describing the volumes above the mentioned level, h_s imposing a coefficient n equal to 1.5 for the rescaled stage-storage capacity curve (referred to the stage-storage capacity curve above the crest level). With this aim, the parameter can be computed by comparing the two functions and imposing that they are equal in a point h_m which is representative of the range of variability of the water level above the crest level.

$$\begin{array}{}\text{(8)}& w_{\mathrm{2}}={\displaystyle \frac{W_{\mathrm{1}}{\left(h_{\mathrm{s}}+h_{\mathrm{m}}\right)}^n-W_{\mathrm{1}}{h}_{\mathrm{s}}^n}{h_{\mathrm{m}}^{\mathrm{1.5}}}}.\end{array}$$

Within the present article, we assumed the parameter h_m was set equal to h_s.

These assumptions allowed deriving a functional relationship between the reservoir inflow and outflow, which can be used to invert the function with respect to the incoming flow and associate a probability with each flow discharged value by exploiting the theory of derived distributions (Benjamin and Cornell, 2014).

Estimation of the event duration

The assumption of a rectangular hydrograph may produce a significant overestimation of the flood volume. Therefore, the parameter t_p should be defined accounting for the real volume associated with a realistic flood hydrograph. In this context, we can rely on the flow duration frequency reduction curve (FDF) proposed by the NERC (Natural Environment Research Council, 1975), which describes the maximum average discharge q(D) as a function of the event duration D:

$$\begin{array}{}\text{(9)}& q\left(D\right)=Q_{\max }{e}^{-\frac{D}{\mathit{\omega }}},\end{array}$$

where ω represents the characterizing basin time response that is frequently associated with the lag time of the river basin.

Adopting the above formulation, Fiorentino (1985) suggested a simple form of synthetic hydrograph redistributing the volume symmetrically with respect to the time of the peak. This leads to the following form of hydrograph:

$$\begin{array}{}\text{(10)}& q\left(t\right)=Q_{\max }{e}^{-\mathrm{2}\frac{\mathrm{|}t\mathrm{|}}{\mathit{\omega }}}.\end{array}$$

Based on the above formulation, it is possible to impose that the duration t_p of the equivalent rectangular event has the same volume of the synthetic hydrograph of Eq. (10) in the temporal window of ω around the peak flow. This led to the following equivalence:

$$\begin{array}{}\text{(11)}& t_{\mathrm{p}}={\displaystyle \frac{\left(e-\mathrm{1}\right)}{e}}\mathit{\omega }\cong \mathrm{0.632}\mathit{\omega }.\end{array}$$

Given the above assumption, the term t_p will be named equivalent event duration from now on.

2.2 Functional relationship between the incoming discharge and the outflow

The possibility to identify the analytical relationship between two processes where one represents the stochastic forcing allows determining the derived probability distribution of the variable. This approach has been used several times for flood maxima (Eagleson, 1972; De Michele and Salvadori, 2002; Gioia et al., 2008), soil moisture (Rodríguez-Iturbe and Porporato, 2007; Manfreda and Fiorentino, 2008), and scour process (Manfreda et al., 2018). In the present case, the methodology has been applied to the laminated flood peak.

With this aim, it is critical to identify the inverse function describing the laminated flood peaks as a function of the maximum floods reaching the detention dam. Equation (6) cannot be inverted in a closed form. Therefore, the function has been approximated using a Taylor expansion of the first order about the point $\mathrm{2}{Q}_{\mathrm{c}}+\frac{W_{\max }}{t_{\mathrm{p}}}$, where $Q_{\mathrm{c}}+\frac{W_{\max }}{t_{\mathrm{p}}}$ represents the incoming discharge that leads to overspilling. This approximation allows defining a closed form of the inverse function, which represents a good approximation for theoretical derivation.

Given the above approximations, it is possible to derive the inverse function of the peak discharge function of the peak outflow. This equation can be obtained mathematically by inverting Eq. (6) and exploiting the parametrization introduced above. The inverse function assumes the following form:

$$\begin{array}{}\text{(12)}& \begin{array}{rl}& Q_{\max }=g^{-\mathrm{1}}\left(Q_{\mathrm{p},\mathrm{out}}\right)\\ & ={\displaystyle \frac{\left(W_{\max }\left(\mathrm{2}{Q}_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)-k_{\mathrm{eq}}{Q}_{\mathrm{c}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)\right)e^{\frac{W_{\max }{t}_{\mathrm{p}}}{k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)}}+k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)Q_{\mathrm{p},\mathrm{out}}{e}^{\frac{t_{\mathrm{p}}}{k}}}{k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)e^{\frac{t_{\mathrm{p}}}{k}}+\left(t_{\mathrm{p}}{W}_{\max }-k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)\right)e^{\frac{W_{\max }{t}_{\mathrm{p}}}{k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)}}}}.\end{array}\end{array}$$

This expression can be used to analytically compute the derived probability distribution of the peak outflow from a detention dam characterized by a storage capacity W_max, an equivalent delay constant k_eq, invested by flood hydrograph of equivalent event duration t_p. With this aim, any probability distribution of the flood peaks can be used given the monotonic nature of the above expression. The expression of the TDD will be (see Benjamin and Cornell, 2014)

$$\begin{array}{}\text{(13)}& f_y\left(y\right)=\left|{\displaystyle \frac{\mathrm{d}{g}^{-\mathrm{1}}\left(y\right)}{\mathrm{d}y}}\right|f_x\left(g^{-\mathrm{1}}\right(y\left)\right),\end{array}$$

where the derivative of $g^{-\mathrm{1}}\left(Q_{\mathrm{p},\mathrm{out}}\right)$ assumes the following form:

$$\begin{array}{}\text{(14)}& \begin{array}{rl}& {\displaystyle \frac{\mathrm{d}{g}^{-\mathrm{1}}\left(Q_{\mathrm{p},\mathrm{out}}\right)}{\mathrm{d}{Q}_{\mathrm{p},\mathrm{out}}}}=\\ & {\displaystyle \frac{k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)e^{\frac{t_{\mathrm{p}}}{k_{\mathrm{eq}}}}}{k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)e^{\frac{t_{\mathrm{p}}}{k_{\mathrm{eq}}}}+\left(t_{\mathrm{p}}{W}_{\max }-k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)\right)e^{\frac{W_{\max }{t}_{\mathrm{p}}}{k_{\mathrm{eq}}\left(Q_{\mathrm{c}}{t}_{\mathrm{p}}+W_{\max }\right)}}}}.\end{array}\end{array}$$

In order to describe the probability distribution of the outflows, we should divide it according to the three potential configurations of the detention dams: (1) undisturbed flow, (2) accumulation of water in the reservoir, and (3) activation of the crest spillway. Based on these assumptions, the probability distribution of the outflow is subdivided into three components and modelled by the following set of equations:

$$\begin{array}{}\text{(15)}& p\left(Q_{\mathrm{p},\mathrm{out}}\right)=\left\{\begin{array}{l}{p}_{Q_{\max }}\left(Q_{\mathrm{p},\mathrm{out}}\right),Q_{\mathrm{p},\mathrm{out}}<Q_{\mathrm{c}}\\ \underset{Q_{\mathrm{c}}}{\overset{\frac{W_{\max }}{t_{\mathrm{p}}}+Q_{\mathrm{c}}}{\int }}{p}_{Q_{\max }}\left(Q_{\mathrm{p},\mathrm{out}}\right)\mathrm{d}q,Q_{\mathrm{p},\mathrm{out}}=Q_{\mathrm{c}}\\ \left|\frac{\mathrm{d}{g}^{-\mathrm{1}}\left(Q_{\mathrm{p},\mathrm{out}}\right)}{\mathrm{d}{Q}_{\mathrm{p},\mathrm{out}}}\right|f_{Q_{\mathrm{p},\mathrm{out}}}\left(g^{-\mathrm{1}}\right(Q_{\mathrm{p},\mathrm{out}}\left)\right),Q_{\mathrm{p},\mathrm{out}}>Q_{\mathrm{c}}.\end{array}\right.\end{array}$$

Equation (15) describes the general form of the probability distribution of the outflow from a detention dam, where the first component coincides with the distribution of the incoming flow as long as it is below the control discharge of the lower opening ($Q_{\mathrm{p},\mathrm{out}}<Q_{\mathrm{c}}$). Assuming that the lower opening is able to control the outflow around Q_c after submergence, there is a mass probability in Q_c depending on the storage volume of the reservoir ($Q_{\mathrm{p},\mathrm{out}}=Q_{\mathrm{c}}$). After these two phases, the outflow is affected by the lamination due to the water volume accumulation above the crest level ($Q_{\mathrm{p},\mathrm{out}}>Q_{\mathrm{c}}$).

3 Applications

3.1 Examples of application of the TDD of the detention basin outflows under different configurations

In order to explore the behaviour of the proposed formulation, we investigated the effects of different types of parametrization on the derived distribution starting from a single distribution of floods. In Fig. 3, the influence of the storage capacity and hydrograph duration on the outflow of the reservoir is shown. We depicted the probability density functions (PDFs) associated with increasing storage capacity obtained by raising the crest level from 2 m up to 8 m (moving top–down in the figure) and considering two distinct equivalent event durations of 30 min and 1 h. As expected, hydrographs with longer duration tend to saturate sooner the water storage capacity of the reservoir, reducing also the lamination effects. On the other hand, the increase in water storage capacity leads to a proportional growth of flood peak mitigation. These graphs describe the behaviour of a reservoir providing an output consistent with the dynamics of the process.

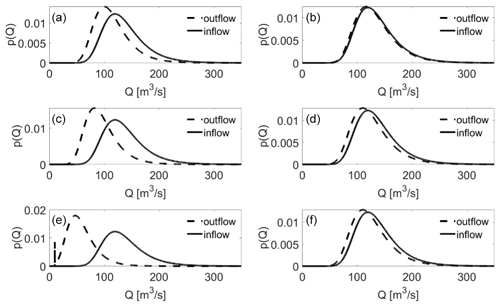
Figure 3 Derived probability density functions of the peak outflow obtained by modifying the height of the spillway crest (h_s) and the equivalent event duration t_p. Graphs on the left (a, c, e) are associated with a duration of half an hour and those on the right (b, d, f) to a duration of 1 h, while elevation of the crest changes between 2 (a, b), 4 (c, d) and 8 m (e, f). Other parameters are α=30 m³/s; β=120 m³/s; b=1 m; d=1 m; n=1.5; $h_{\mathrm{f}}=l_{\mathrm{f}}+d/\mathrm{2}=\mathrm{0.5}$ m; h_s=2; μ_f=0.85; μ_s=0.385; L=5 m.

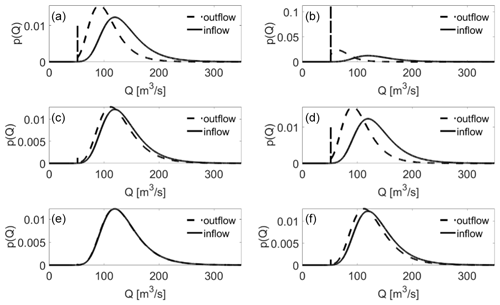
Figure 4 Derived probability density functions of the outflow associated with different equivalent event durations, t_p, using two different coefficients of the stage-storage capacity curve, w₁, which was set equal to 5000 in (a), (c), and (e), while it assumes values of 10 000 m³ in (b), (d), and (f). Event duration changes between 0.5 (a, b), 1 (c, d), and 2 h (e, f). Other parameters are α=30 m³/s; β=120 m³/s; b=4 m; d=2 m; n=1.5; $h_{\mathrm{f}}=l_{\mathrm{f}}+d/\mathrm{2}$; h_s=4; μ_f=0.85; μ_s=0.385; L=6 m.

In Fig. 4, we modified the coefficient of the stage-storage capacity curve, w₁, using the values of 5000 and 10 000 and explored equivalent event durations ranging from half an hour to 2 h. Graphs display how the PDFs of outflows are altered by the presence of a dam with these characteristics. In the present example, the impact of the lower opening can be better appreciated with a mass probability around Q_c that is equal to 52 m³/s. It must be clarified that the cross-section of the opening has been increased in this second example on purpose to emphasize its impact on the proposed mathematical scheme.

3.2 Testing the reliability of the proposed method

In the current work, a numerical simulation of flood mitigation through a detention basin with the characteristics reported in Fig. 1 was carried out using the same forcing adopted for the proposed TDD. Therefore, we adopted the Gumbel distribution as the reference distribution to generate random values of discharge and numerically simulate the dynamics of the detention dam and its peak outflows. It must be clarified that any probability distribution of floods (e.g. generalized extreme value, three-parameter log-normal, generalized logistic and Gumbel distributions) can be applied. An example of the numerical simulation is given in Fig. 2 with the consequent attenuation of the hydrograph due to the detention dam.

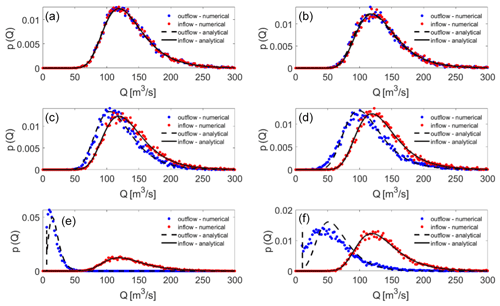
Figure 5 Comparison between derived probability density functions of the outflow (continuous black line for the inflow and dashed black line for the outflow) and the empirical PDFs obtained via numerical hydraulic simulation (red dots for inflow and blue dots for outflow). Graphs provide the following parameterizations: (a) w₁=2000; h_s=4 m; (b) w₁=1500; h_s=9 m; (c) w₁=5000; h_s=4 m; (d) w₁=3000; h_s=10 m; (e) w₁=40 000; h_s=4 m; (f) w₁=6000; =10 m. Remaining parameters are α=30 m³/s; β=120 m³/s; b=1 m; d=1 m; n=1.9; $h_{\mathrm{f}}=d/\mathrm{2}$; μ_f=0.85; μ_s=0.385; L=4 m; t_p=2 h.

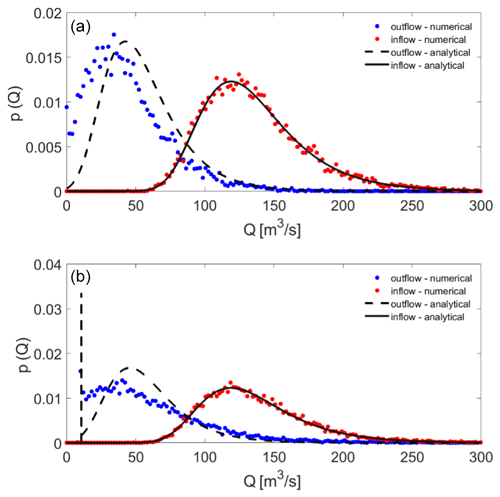
Figure 6 Comparison between derived probability density functions of the outflow (continuous black line for the inflow and dashed black line for the outflow) and the empirical PDFs obtained via numerical hydraulic simulation (red dots for inflow and blue dots for outflow) obtained by assuming the absence (a) or the presence (b) of the low-level opening. Remaining parameters are α=30 m³/s; β=120 m³/s; w₁=6500; h_s=10 m; b=1 m; d=1 m; n=1.9; $h_{\mathrm{f}}=d/\mathrm{2}$; μ_f=0.85; μ_s=0.385; L=4 m; t_p=2 h.

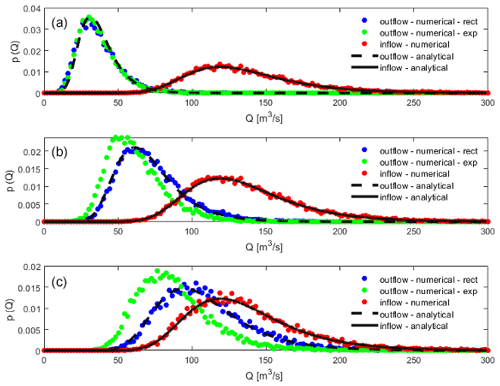
Figure 7 Comparison between derived probability density functions of the outflow (continuous black line for the inflow and dashed black line for the outflow) and the empirical PDFs obtained via numerical hydraulic simulation (red dots for inflow, blue dots for outflow obtained by incoming rectangular hydrographs and green dots for outflow obtained by incoming exponential hydrographs), assuming three equivalent event durations t_p of half an hour (a), 1 h (b) and 2 h (c). Remaining parameters are α=30 m³/s; β=120 m³/s; w₁=5000; h_s=4 m; b=1 m; d=1 m; n=1.9; $h_{\mathrm{f}}=d/\mathrm{2}$; μ_f=0.85; μ_s=0.385; L=3 m.

The numerical simulation was carried out with the main scope of testing the theoretically derived probability distribution of laminated peak flows and also quantifying the impact of the approximations adopted to obtain a closed form of the solution. Therefore, the comparison of the theoretically derived distribution and numerical outflows helps understand the reliability of the proposed methodology. Results are given in the following graphs.

Figure 5 provides a comparison of different PDFs obtained, modifying the maximum water storage capacity of the dam and its height. It can be noted that the values of the probability distribution replicate fairly well those obtained with the numerical simulations. The approximation of a fixed discharge from the submerged opening induces a small dispersion of values around the control value of discharge, Q_c, that the theoretical probability distribution is not able to capture. Moreover, the adopted approximations slightly overestimate the outflows which can be interpreted as a safety approximation for flood mitigation planning.

Comparing the different PDFs, it should be clarified that the parameters have been changed, looking for combinations of dam heights, h_s, and coefficient w₁ leading to similar water storage capacity on each row. This allows demonstrating that it is much more effective to increase the area flooded by the reservoir (the parameter w₁ represents the rate of increase of the water storage with the water level) rather than increase the height of the dam.

The last analysis performed is given in Fig. 6, where we tested the performances of the TDD assuming the lower opening closed. Such a condition may be representative of an ordinary dam with an assigned flood retention volume or used for water supply purposes, where the volume above the crest spillway leads to a lamination of floods. This configuration has been compared with the scheme described in the previous sections that includes the presence of the lower opening. The difference between the two configurations is given in Fig. 6a (closed opening) and b (lower opening active). The two graphs show the ability of the mathematical formulation to properly interpret also the present configuration, offering a wide spectrum of potential applications in hydraulic design.

Finally, in order to test the impact of the assumption of rectangular inflow hydrograph, we also compared the results of a numerical simulation where the hydrograph is assumed to be a symmetric exponential one according to the expression given in Eq. (11). The comparison is given in Fig. 7 for three different equivalent event durations. It can be appreciated how the use of an equivalent event duration produced probability distributions of the outflow that look very similar to those obtained with a symmetric exponential hydrograph. It must also be underlined that with the increase of the duration of the event such an approximation tends to deteriorate the result of the proposed model.

4 Conclusion

The present article introduces a new formulation useful to quantify the impact of detention dams on the probability distribution of floods. We must acknowledge that the formulation was obtained with several simplifying assumptions that include the shape of the incoming hydrograph, the approximation used to interpret the flow through the lower opening, and the approximation of a linear reservoir for the flow above the crest level. Summing all these, it is really satisfying to see that the obtained formulation can interpret the dynamics of such hydraulic infrastructures fairly well, providing an analytical description of the impact of artificial reservoirs on flood dynamics. This may be extremely useful in properly addressing the effects of water infrastructures on floods. The TDD can be used for detention dams, but the formalism can also be applied to ordinary dams just setting the control discharge to zero and assigning a given value of the water level in the dam. Therefore, the formalism is versatile and can be applied in different contexts. The strongest assumption is represented by the rectangular hydrograph which can be realistic for small river basins characterized by relatively short concentration time. With the aim of minimizing the impact of such a choice, we adopted an equivalent event duration in the formulation that allowed accounting for the flood variability during a specific event. However, this assumption may become limitative in large river basins where a hydrograph evolves over large areas and its shape is also not simple to be predicted. In fact, large river basins may display complex hydrographs with multiple peaks that require a specific approach.

The proposed method may be used in some contexts such as the projects and design of small lamination dams and detention dams in small river basins. For instance, there are several river basins along the coastline that drain a high amount of water in short durations, affecting cities and towns developed along the waterfront. These areas are typically exposed to frequent flood events that may impose the need to properly identify potential solutions for flood mitigation.

The scheme can be used to carry out preliminary dimensioning of these structures and eventually could be coupled with other tools to identify optimal configurations for flood mitigation. Moreover, the scheme can be applied to any probability distribution of floods, including the case of floods that are already subject to laminations allowing the description of a scheme of nested dams.

This topic is still under investigation, and its study will be applied to identify optimal solutions in flood control systems quantifying the impact of structure on the full spectra of floods.

Appendix A: Notations

α [–]	Scale parameter of Gumbel distribution
β [–]	Location parameter of Gumbel distribution
μf [–]	Coefficient of discharge of the submerged low-level opening
μs [–]	Coefficient of discharge of the crest spillway
A [m2]	Area of the low-level opening
b [m]	Width of the low-level opening rectangular section
d [m]	Height of the low-level opening
g [m/s2]	Acceleration due to gravity
h [m]	Variable water level within the detention basin
hm [m]	Mean of water levels over the spillway crest
hs [m]	Height of the spillway crest
hf [m]	Height of the barycentre of the low-level opening
k [s]	Storage coefficient of the linear reservoir method
keq [s]	Equivalent delay constant of the conceptual reservoir associated with the outflow
L [m]	Effected crest length
n [–]	Exponent of the stage-storage capacity curve
p(Q) [–]	Probability density function of outflows
lf [m]	Height of the low-level opening
Qc [m3/s]	Design outflow from the low-level opening
Qin [m3/s]	Inflow in the detention basin
Qmax [m3/s]	Peak flow incoming in the detention basin
Qout [m3/s]	Outflow from the detention basin
Qp,out [m3/s]	Peak outflow from the detention basin
t [s]	Time
D [s]	Flood event duration
tfilling [s]	Time after which the crest spillway starts functioning
tp [s]	Equivalent flood duration
ω [s]	Lag time of the river basin
Wmax [m3]	Water storage capacity at the crest level
W [m3]	Variable storage capacity of the detention basin
w1 [m3−n]	Parameter of the stage-storage capacity curve
w2 [m${}^{\mathrm{3}/\mathrm{2}}$]	Parameter of the equivalent stage-storage capacity curve