River runoff in Switzerland in a changing climate – runoff regime changes and their time of emergence

Assessments of climate change impacts on runoff regimes are essential to climate change adaptation and mitigation planning. Changing runoff regimes and thus changing seasonal patterns of water availability strongly influence various economic sectors such as agriculture, energy production, and fishery and also affect river ecology. In this study, we use new transient hydrological scenarios driven by the most up-to-date local climate projections for Switzerland, the Swiss Climate Change Scenarios. These provide detailed information on changes in runoff regimes and their time of emergence for 93 rivers in Switzerland under three Representative Concentration Pathways (RCPs): RCP2.6, RCP4.5, and RCP8.5. These transient scenarios also allow changes to be framed as a function of global mean temperature.

The new projections for seasonal runoff changes largely confirm the sign of changes in runoff from previous hydrological scenarios with increasing winter runoff and decreasing summer and autumn runoff. Spring runoff is projected to increase in high-elevation catchments and to decrease in lower-lying catchments. Despite the increases in winter and some increases in spring, the annual mean runoff is projected to decrease in most catchments. Compared to lower-lying catchments, runoff changes in high-elevation catchments (above 1500 m a.s.l.) are larger in winter, spring, and summer due to the large influence of reduced snow accumulation and earlier snowmelt and glacier melt. The changes in runoff and the agreement between climate models on the sign of change both increase with increasing global mean temperatures and higher-emission scenarios. This amplification highlights the importance of climate change mitigation.

The time of emergence is the time when the climate signal emerges significantly from natural variability. Under RCP8.5, times of emergence were found early, before the period 2036–2065, in winter and summer for catchments with mean altitudes above 1500 m a.s.l. Significant changes in catchments below 1500 m a.s.l. emerge later in the century. Not all catchments show significant changes in the distribution of seasonal means; thus, no time of emergence could be determined in these catchments. Furthermore, the significant changes of seasonal mean runoff are not persistent over time in some catchments due to nonlinear changes in runoff.

1 Introduction

Anthropogenic climate change is certain to affect regional and local hydrology (IPCC, 2013, 2014a, b). Major research efforts have enabled more precise and more reliable projections of regional and local temperature and precipitation changes (CH2018, 2018). The new Swiss Climate Change Scenarios (CH2018) are the result of a large modeling effort to downscale regional climate projections for Switzerland to local scales (CH2018, 2018). Warming in Switzerland is projected to be higher than the global mean warming. Without major mitigation efforts, mean temperatures are projected to increase by up to 6.8 ^∘C by end of the 21st century in Switzerland (CH2018, 2018). This large increase in temperatures is accompanied by changes in many other hydrologically relevant variables such as precipitation amounts, precipitation type, and glacier volumes. The projected combination of increasing temperatures, changing precipitation patterns, retreating glaciers, and changes in snowpack will have a potentially strong impact on runoff regimes. Runoff regimes reflect the integral response in time and space of the hydrological conditions within a catchment and hence its water supply. Understanding and assessing changes in runoff regimes are crucial for many economic sectors, such as agriculture, fishery, hydropower generation, and tourism, and for the ecology within and around the rivers. Assessments of climate change impacts on river runoff are therefore particularly important for decision makers in adaptation planning and can also provide a basis for mitigation policies.

Several studies on the impacts of climate change on hydrology in Switzerland have been conducted in recent years. These have focused on changes in runoff regimes (Horton et al., 2006; Köplin et al., 2012, 2014; Addor et al., 2014; Milano et al., 2015), on changes in low flows (Jenicek al., 2018; Brunner et al., 2019a, b), on changes in high flows (Keller et al., 2018; Brunner et al., 2019a), and on the effects of retreating glaciers for rivers (Huss et al., 2008, 2014; Farinotti et al., 2012; Finger et al., 2012; Fatichi et al., 2015; Etter et al., 2017). Studies on climate change impacts on various aspects of the hydrology in the Alpine area have also been carried out in Austria (e.g., Weber et al., 2010; Blöschl et al., 2011; Prasch et al., 2011; Tecklenburg et al., 2012; Goler et al., 2016; Wijngaard et al., 2016; Hanzer et al., 2018), in Italy (e.g., Groppelli et al., 2011), in Germany (e.g., Hattermann et al., 2015; Nilson et al., 2014), and in France (e.g., Ruiz-Villanueva et al., 2015; Vidal et al., 2016). Most of these studies are case studies or focus on specific aspects of hydrology.

Previous studies have focused on changes in runoff regimes and included many catchments with diverse properties in Switzerland (Horton et al., 2006; Koeplin et al., 2012; Köplin et al., 2014; Addor et al., 2014). Köplin et al. (2012) found a shift in seasonality with increasing winter runoff, decreasing summer runoff, unchanging annual mean runoff for lower-lying catchments, and increasing annual mean runoff for high-Alpine catchments. However, these studies are based either on older climate model generations or on climate simulations downscaled with a delta change approach. The delta change method is based on climate simulations downscaled for 30-year periods both in the reference period and in future periods and does not provide continuous transient simulations for the whole 21st century. Therefore, this approach does not simulate daily to interannual variability (Bosshard et al., 2011). More sophisticated downscaling approaches have been developed, such as quantile mapping (Teutschbein and Seibert, 2012; Gudmundsson et al., 2012). They correct not only for the mean bias but also for the full distribution; they are applicable to long-term climate simulations and allow transient scenarios to be established. Using quantile mapping as a downscaling approach can intensify and increase the number of projected extremes (Rössler et al., 2019). Therefore, the present study uses the Hydro-CH2018-Runoff ensemble (dataset: Muelchi et al., 2020, 2021) run with the most up-to-date local climate change scenarios for Switzerland (CH2018). These scenarios used the quantile mapping approach to downscale the coarse climate model output. The Hydro-CH2018-Runoff ensemble includes transient daily simulations from 1981 to 2099 for 93 catchments in Switzerland and three Representative Concentration Pathways (RCPs): RCP2.6, RCP4.5, and RCP8.5. The new ensemble enables detailed quantification of changes in runoff regimes. In this study, we investigate changes in runoff regimes under a range of RCP scenarios. These 119-year continuous daily runoff simulations enable the estimation of the time of emergence (Giorgi and Bi, 2009; Leng et al., 2016) of those changes. The time of emergence is the time when the climate signal emerges significantly from natural variability. It is of particular importance to the question of how much time is left for adaptation planning. In this study, we analyze not only the time of emergence but also the evolution of time of emergence over time. Moreover, the ensemble allows for the quantification of changes at different global warming levels; these warming levels are particularly relevant for policymakers (e.g., James et al., 2017). The framing of the results as a function of global mean temperature change rather than time enables a direct link to the Paris Agreement target and indicates the changes to be expected in a +2 ^∘C world and the consequences of missing this target. In this study, we assess changes for global warming levels of +1.5, +2, and +3 ^∘C.

2 Data

For this study, we use the Hydro-CH2018-Runoff ensemble of daily discharge simulations for 93 medium-sized (catchment size between 14–1700 km²) catchments in Switzerland (Fig. 1; Muelchi et al., 2020, 2021). The Precipitation-Runoff-Evapotranspiration HRU-related Model (PREVAH) hydrological modeling system (Viviroli et al., 2009) was used for simulating the hydrological response to the climate change scenarios. PREVAH is a semidistributed model based on hydrological response units and includes submodules to account for important hydrological processes related to snow, glacier, and soil moisture dynamics and evapotranspiration. PREVAH was calibrated and validated for each catchment (for more details, see Muelchi et al., 2021). The calibrated parameters were kept constant for the simulation of runoff under climate change, and land use was held unchanged for nonglaciated catchments. The minor impact of land use changes on changes in the runoff regime for Switzerland was assessed by Köplin et al. (2014). For glaciated catchments, glacier extents were updated every 5 years according to the glacier projections by Zekollari et al. (2019), which are based on the same climatic dataset. The land use of areas from which glaciers disappear was replaced by rock for areas above 3000 m a.s.l. and by bare soil for areas below 3000 m a.s.l.

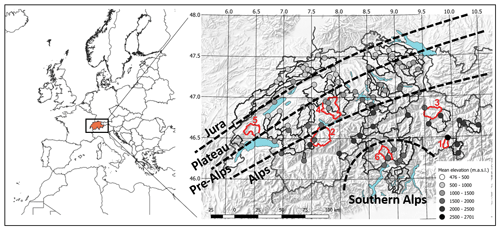
Figure 1 Overview of the study region and the location of the gauging stations in Switzerland. Shading indicates the mean elevation of the respective catchment. Red contours indicate the six example catchments: Rosegbach–Pontresina (1), Kander–Hondrich (2), Plessur–Chur (3), Emme–Emmenmatt (4), Venoge–Ecublens (5), and Verzasca–Lavertezzo (6).

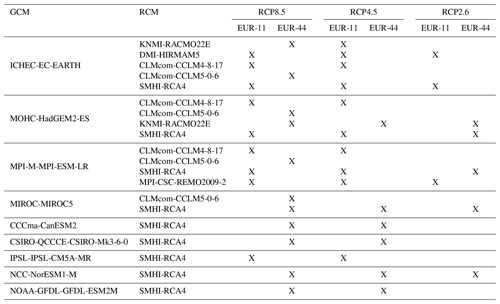
The meteorological input used for the simulations comes from the latest Swiss Climate Change Scenarios CH2018 (CH2018, 2018). The CH2018 scenarios used EURO-CORDEX simulations (Jacob et al., 2014; Kotlarski et al., 2014) and applied statistical downscaling (quantile mapping; Teutschbein and Seibert, 2012; Gudmundsson et al., 2012) on chains of global circulation models and regional climate models (GCM–RCM chains). The results consist of gridded high-resolution (2 km×2 km) daily temperature and precipitation data for Switzerland for the period 1981–2099. This dataset was then used to drive the hydrological model, resulting in the Hydro-CH2018-Runoff ensemble, which comprises daily runoff time series for 1981–2099 for each of the GCM–RCM chains. The Hydro-CH2018-Runoff ensemble contains simulations for three RCPs: 8 simulations under RCP2.6, 16 simulations under RCP4.5, and 20 simulations under RCP8.5. The GCM–RCM chains and their underlying emission scenarios used for this study are listed in Table 1. The Hydro-CH2018-Runoff ensemble comprises transient long-term daily runoff projections for Switzerland for the period 1981–2099. These transient simulations incorporate the daily to interannual climate variability. Changes in runoff constrained by the different warming levels were analyzed using the global mean temperatures of the driving GCMs (CMIP5; Taylor et al., 2012).

Table 1 List of GCM–RCM chains used, their initial resolution, and the RCP available.

3 Methods

3.1 Study area

The study area consists of 93 catchments distributed over Switzerland (Fig. 1) and covering a wide range of catchment characteristics with an average catchment size of 314 km² (from 14 to 1700 km²) and a mean altitude of 1344 m a.s.l. (range in mean altitude: 476–2700 m a.s.l.). Of the 93 catchments, 22 are glaciated with a modeled degree of glaciation varying between 1 % and 29 % (Fig. S1 in the Supplement). The present runoff regimes range from glacier-fed catchments in high-Alpine areas, mainly snow-driven catchments (mean altitude above 1550 m a.s.l.) in the Alps and pre-Alps, to rain-fed catchments predominant in the Swiss Plateau and at lower elevations in the southern part of Switzerland. Six catchments (highlighted in Fig. 1) are used as example catchments representing typical runoff regimes: Rosegbach, highly glaciated (29 %); Kander, slightly glaciated (5 %); Plessur, high-Alpine snow influenced; Emme, pre-Alpine rain and snow influenced; Venoge, lowland rain dominated; and Verzasca, southern Alpine rain and snow dominated. An overview of the catchment characteristics (Table S1 in the Supplement), the degree of glaciation (Fig. S1), and the fraction of precipitation falling as snow (Fig. S2) can be found in the Supplement.

3.2 Determining changes in seasonal and annual mean runoff

The simulations were analyzed using annual and seasonal changes of runoff under the three RCPs. The seasons were defined as winter (December–February), spring (March–May), summer (June–August), and autumn (September–November). All changes were specified for 30-year periods to remove interannual variability. The reference period covers the years 1981–2010 and was compared with the far future period 2070–2099. The median among all simulations within an RCP pathway was considered the best estimate. To obtain an indication of the robustness of the estimation, the changes were highlighted when at least 90 % of the simulations showed the same direction of change, whether positive or negative. This criterion corresponds to “very likely” in the terminology of the Intergovernmental Panel on Climate Change (IPCC) that the runoff changes are either positive or negative (Mastrandrea et al., 2010). The changes in seasonal and annual runoff were also analyzed as a function of the mean elevation of the catchments to show the elevation dependence of runoff responses. Other elevation-related characteristics of a catchment might have been used. However, a study by Köplin et al. (2012) showed that catchment responses to climate change in Switzerland can be directly linked to mean altitude.

For the analysis of changes in the runoff regime, monthly means for 30-year periods were calculated with the median representing the best estimate and with the uncertainty band showing the full range among all models within an RCP. To simplify the interpretation of the results, this article focuses only on changes up to the period 2070–2099 under RCP8.5 and RCP2.6. These two emission scenarios provide the broadest range of changes available from the full Hydro-CH2018-Runoff ensemble. Results for the near-future period of 2045–2074 and for RCP4.5 can be found in the Supplement.

3.3 Determination of the time of emergence of seasonal and annual runoff changes

The time of emergence (Giorgi and Bi, 2009; Leng et al., 2016) indicates the time when significant changes in the distribution of seasonal and annual means emerge from natural variability. The Kolmogorov–Smirnov test was used to test whether two 30-year samples of seasonal or annual means are drawn from the same distribution. This test was conducted on the distributions of moving 30-year windows and the distribution of the reference period. The Kolmogorov–Smirnov test procedure was also used in previous studies (e.g., for precipitation Mahlstein et al., 2011; Gaetani et al., 2020), but other definitions of time of emergence also exist. Although Mahlstein et al. (2011) did not find significant differences from other definitions, Gaetani et al. (2020) found that the Kolmogorov–Smirnov testing procedure results in a more robust and earlier time of emergence. The testing was performed for each simulation under RCP8.5 and each catchment separately. Constraining the analysis to the RCP8.5 ensemble was motivated by the sufficiently large number of simulations, 20, within the ensemble. The time of emergence was then defined by following the procedure used in Mahlstein et al. (2011) and refers to the last year of the 30-year moving window in which the Kolmogorov–Smirnov test was rejected for the first time at 95 % significance. We then considered the significance of changes in the seasonal and annual mean when at least 66 % of the models detect a significant change in the same 30-year window. Sixty-six percent corresponds to the threshold referred to as “likely” in the IPCC terminology (Mastrandrea et al., 2010). Because changes in runoff may not be linear over time, the time of emergence may not be stable after the first detection. Even though changes in seasonal and annual runoff are tested as significant in one period, they may not be significant in all subsequent periods (e.g., due to nonlinear effects in snowmelt or glacier melt contributions). Therefore, we also analyzed the temporal evolution of rejections of the null hypothesis for the Kolmogorov–Smirnov test (p values smaller than 0.05).

3.4 Stratification of seasonal and annual runoff changes by increases in global mean temperature

For the analysis of runoff changes as a function of global mean temperature change, temperature targets were defined at +1.5, +2, and +3 ^∘C with respect to the preindustrial state. Because the temperature targets are defined with respect to the preindustrial state, the warming observed between the preindustrial state (1864–1900) and the reference period (1981–2010) was subtracted from the temperature targets. The observed warming is estimated to be 0.6 ^∘C; thus, the remaining global warming for the 1.5, 2, and 3 ^∘C temperature targets is 0.9, 1.4, and 2.4 ^∘C, respectively (Morice et al., 2012; for technical details, see CH2018, 2018). For each of the driving GCMs used in the Hydro-CH2018-Runoff ensemble for RCP8.5, we computed differences in moving 30-year averages of global mean temperatures compared to the reference period. The 30-year windows when global mean temperature change exceeds +0.9, +1.4, and +2.4 ^∘C were selected for each GCM. Subsequently, the seasonal and annual changes in runoff were extracted for each of the time periods and the driving GCM in the GCM–RCM combination. Again, catchments with robust signs were highlighted if at least 90 % of simulations agreed on the direction of change.

4 Results

4.1 Changes in seasonal and annual mean runoff for Switzerland

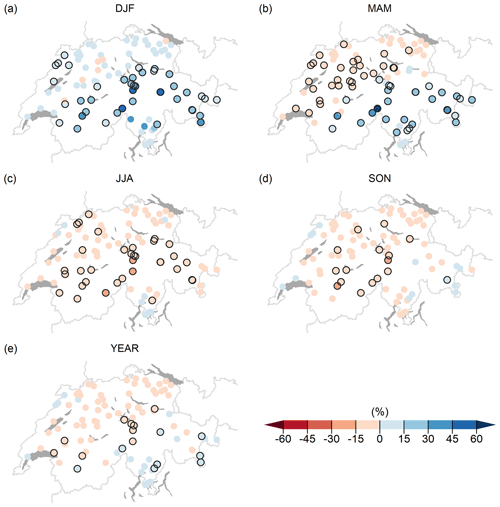
Changes in the multimodel median of seasonal and annual mean runoff by end of the century (2070–2099) are shown in Fig. 2 for RCP8.5 and in Fig. 3 for RCP2.6 (see Fig. S3 for RCP4.5 and Figs. S4–S6 for the period 2045–2074). Highlighted catchments show changes where at least 90 % of the models agree on the sign of change.

Figure 2 Multimodel median of seasonal and annual mean changes of runoff under RCP8.5 by 2070–2099 for winter (a), spring (b), summer (c), autumn (d), and annual means (e). Black circles indicate catchments whose direction of change agrees across at least 90 % of the models.

Figure 3 Equivalent to Fig. 2 but with projections for RCP2.6.

4.1.1 Changes in winter runoff for Switzerland

In winter, all catchments show positive mean runoff changes under RCP8.5 by the end of the century (Fig. 2a) compared to the reference period. The mean runoff changes range from +2 % to +221 % with larger changes in higher-elevation catchments. The mean change among all catchments is +48 %. In all, 84 out of 93 catchments show good agreement (>=90 %) on the sign of change. Under RCP2.6, 87 out of 93 catchments show positive changes, with mean runoff changes across all catchments of +13 % (Fig. 3a). However, only 41 catchments show robust model agreement on the sign of change. The range among the catchments is between −3 % and +58 %, again with stronger changes in the mountainous areas.

4.1.2 Changes in spring runoff for Switzerland

In spring, both positive and negative changes are found in mean runoff (Figs. 2b and 3b). The mean change across all catchments is +9 % under RCP8.5 (Fig. 2b). Although most of the lower catchments show a decrease in runoff (up to −21 %), the higher-elevation catchments exhibit an increase (up to +166 %) under RCP8.5. The strong increase in spring runoff is mainly found in the highest-elevation catchments (Fig. 2b), where snowmelt is increased due to higher temperatures that shift the snowmelt season from early summer to spring. However, only 34 out of 93 catchments exhibit robust changes across the climate models. Compared to the high-emission scenario, the changes in the low-emission scenario (RCP2.6) tend to be more moderate (Fig. 3b). Under RCP2.6, the changes range from −15 % to +70 % with a slightly positive mean (+3 %) across all catchments. Fifty-eight catchments show robust changes in spring mean runoff under RCP2.6. Some catchments show negative changes under RCP2.6 but positive changes under RCP8.5. This transition from negative to positive changes is also found in RCP4.5 (Fig. S3).

4.1.3 Changes in summer runoff for Switzerland

In summer and under RCP8.5, all catchments show a decrease in mean runoff, ranging from −16 % to −59 % with mean change of −35 % across all catchments (Fig. 2c). Again, more pronounced negative changes are found in higher-elevation catchments, where summer runoff is projected to decrease by up to half of the runoff of the reference period. All catchments except one agree on the sign of change among the climate models. Under RCP2.6, the signs and magnitude of the changes are less clear (Fig. 3c). The average change across all catchments is negative (−6%), but mean runoff change ranges from −26 % to +4 %. Just 9 catchments show positive but non-robust mean runoff changes in summer, whereas 34 catchments yield robust negative signs.

4.1.4 Changes in autumn runoff for Switzerland

In autumn, all catchments but one show decreasing runoff under RCP8.5 (Fig. 2d). Changes range from −36 % to +4 % with an average change of −19 % across all catchments. More than 50 % of the catchments reveal robust changes. In contrast to the changes in summer, the autumn runoff tends to decrease more strongly in the lower-lying catchments than in the higher-elevation catchments. Under RCP2.6, the changes are much smaller than under RCP8.5, with an average change of −5 % (ranging from −20 % to +10 %; Fig. 3d). The changes are also less robust, with only 17 catchments showing good agreement on the sign of change.

4.1.5 Changes in annual runoff for Switzerland

Despite the increases in winter and partial increases in spring, the annual runoff is projected to decrease by 8 % (range: −23 % to +4 %) under RCP8.5 (Fig. 2e) and by 2 % (range: −13 % to +11 %) under RCP2.6 (Fig. 3e) on average. Whereas 82 out of 93 catchments show negative changes under RCP8.5, only 65 catchments exhibit negative changes under RCP2.6. However, the robustness of the sign of change in the annual mean runoff is weaker than that of the seasonal mean changes.

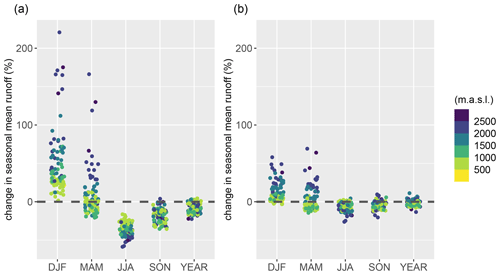
4.1.6 Elevation dependence of mean seasonal and annual changes in runoff

Considering the results above, changes in seasonal and annual mean runoff are heavily dependent on the mean elevation of the catchment. This dependence is highlighted in Fig. 4, where changes in runoff are plotted against mean altitude of the catchments. The higher-elevation catchments generally show larger changes in winter, spring, and summer than the lower-elevation catchments. For autumn and annual runoff, no distinct pattern can be seen. Under RCP8.5 and for catchments with mean altitude below 1500 m a.s.l., the average change is +27 % in winter, −5 % in spring, −31 % in summer, −21 % in autumn, and −8 % in the annual mean (Fig. 4a). For catchments with mean elevation above 1500 m a.s.l., runoff changes on average by +77 % in winter, by +28 % in spring, by −41 % in summer, by −15 % in autumn, and by −9 % in the annual mean. However, the changes in the higher-elevation catchments are less pronounced under RCP2.6, with an average change in catchments below 1500 m a.s.l. of +5 % in winter, −6 % in spring, −4 % in summer, −6 % in autumn, and −4 % in annual mean runoff (Fig. 4b). In higher-elevation catchments (>1500 m a.s.l.), the mean changes under RCP2.6 amount to +24 % in winter, +16 % in spring, −9 % in summer, −4 % in autumn, and −0.6 % across the year.

Figure 4 Elevation dependence of the multimodel median (dots) of seasonal and annual mean changes of runoff by 2070–2099 under RCP8.5 (a) and RCP2.6 (b). Colors indicate the mean elevation of the 93 catchments.

4.2 Changes in the runoff regime of six representative catchments

Changes in runoff regime for six example catchments representing typical runoff regime types in Switzerland are depicted in Fig. 5. These are the catchments highlighted in Fig. 1: Rosegbach–Pontresina, Kander–Hondrich, Plessur–Chur, Emme–Emmenmatt, Venoge–Ecublens, and Verzasca–Lavertezzo. The runoff regimes with absolute monthly mean runoff presented in this chapter help to interpret the relative changes of seasonal and annual runoff means discussed in Sect. 4.1. Figures with Pardé coefficients and runoff regimes for each RCP separately can be found in the Supplement (Figs. S7–S11).

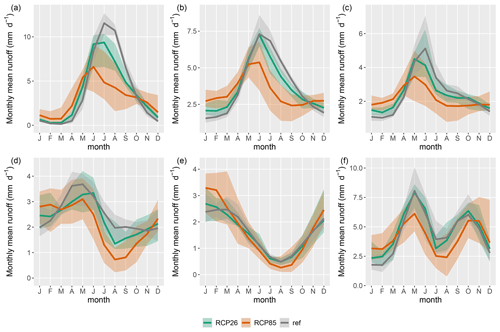
Figure 5 Runoff regimes for the six representative catchments: Rosegbach (a), Kander (b), Plessur (c), Emme (d), Venoge (e), and Verzasca (f). Thick lines represent the multimodel median for the reference period (grey), for 2070–2099 under RCP2.6 (turquoise), and for 2070–2099 under RCP8.5 (orange). Shading shows the full model range for each RCP.

4.2.1 Highly glaciated catchment: Rosegbach–Pontresina

The glaciated catchment, Rosegbach–Pontresina, exhibits a pronounced decrease in July, August, and September (−3.1 to −6.7 mm d⁻¹) and a small increase in winter runoff (+0.5 to +1 mm d⁻¹) under RCP8.5 (Fig. 5a). Monthly mean runoff does not change significantly in October. The typical glacier runoff regime with low flows in winter and peak flows in summer in the reference period changes to a nival regime with a peak runoff shifted from July or August to June under RCP8.5 (Fig. 5a). Although there are large relative (+200 %) increases in the winter months, the contribution of winter runoff to the total runoff remains small. The mean runoff between June and September drops dramatically due to the absence of snowmelt and glacier melt contributions. However, runoff in late spring and summer remains the major contributor to the annual volume. Under RCP2.6, the changes for winter, spring, and autumn runoff are small (+0.1 to +0.7 mm d⁻¹). For July, August, and September, the runoff decreases significantly (−1.7 to −3.5 mm d⁻¹), and the summer peak shifts from July or August to June or July. The change in summer runoff under RCP2.6 is approximately halved compared to the changes under RCP8.5.

4.2.2 Slightly glaciated catchment: Kander–Hondrich

A similar behavior with a shift of the peak in the runoff regime from summer to late spring or early summer is also found for the runoff regime in the slightly glaciated catchment, Kander–Hondrich, under RCP8.5 (Fig. 5b). The regime is characterized by a peak in early summer runoff in the reference period. Under RCP8.5, the summer and early autumn runoff (July–September) decreases significantly (−1.8 to −3.2 mm d⁻¹), whereas winter runoff increases (+0.8 to +1.3 mm d⁻¹). This leads to a flattening of the runoff regime curve, resulting in similar contributions of winter and summer runoff to the annual volume. Under RCP2.6, there is also a decrease in July, August, and September (−0.7 to −1.1 mm d⁻¹) but less pronounced than the decrease under RCP8.5.

4.2.3 Nival catchment: Plessur–Chur

The nival regime of the river Plessur–Chur shows a shift in peak flow from June to May under both emission scenarios (Fig. 5c). Due to the increase in winter months (+0.4 to +0.9 mm d⁻¹) and decrease in summer runoff (−0.9 to −2.1 mm d⁻¹), the regime curve flattens under RCP8.5. The results also show increasing winter runoff and decreasing summer runoff under RCP2.6 but far less pronounced than under RCP8.5.

4.2.4 Nival-to-pluvial catchment: Emme–Emmenmatt

In the reference period, the runoff regime in the nival-to-pluvial catchment, Emme–Emmenmatt, shows a peak in spring and early summer due to snowmelt and stable mean runoff from August to February (Fig. 5d). By end of the century under RCP8.5, the peak runoff in spring almost disappears. The runoff decreases strongly in the summer months (−0.7 to −1.3 mm d⁻¹) and less in the autumn months (−0.1 to −1.2 mm d⁻¹).

4.2.5 Pluvial catchment: Venoge–Ecublens

The shape of the runoff regime curve for the pluvial river Venoge–Ecublens remains the same for the reference period and the two emission scenarios with higher runoff in winter and lower runoff in summer (Fig. 5e). Although the regime changes only marginally under RCP2.6, the amplitudes under RCP8.5 become more distinct, with higher winter runoff (+0.3 to +0.9 mm d⁻¹) and lower summer runoff (−0.2 to −0.3 mm d⁻¹) than in the reference period. However, in comparison to other catchments, this change is smaller.

4.2.6 Southern Alpine catchment: Verzasca–Lavertezzo

The southern Alpine catchment, Verzasca–Lavertezzo, shows a two-peaked runoff regime, with a first runoff peak in late spring and a second runoff peak in autumn in the reference period (Fig. 5f). This pattern is still present at the end of the century under both scenarios. However, the amplitudes of the peaks are less pronounced under RCP8.5 because of increasing winter runoff and decreasing spring (−0.2 to −1.9 mm d⁻¹) and summer (−1.4 to −1.7 mm d⁻¹) runoff.

Summarizing the differences under RCP8.5 and RCP2.6 shows that the sign of change under RCP2.6 is equal to the sign under RCP8.5 in almost all months and catchments. Comparisons with RCP4.5 (see Fig. S5) show that the magnitude of changes increases with the strength of the emission scenario and the distance in time. Also, the model agreement on the direction of change among the climate models is weaker under the low-emission scenario, RCP2.6, than under RCP8.5.

4.3 Time of emergence of changes in the seasonal and annual means

The time of emergence, when at least 66 % of the models under RCP8.5 agree on significant changes in the distribution of seasonal and annual means, is depicted in Fig. 6. In winter, 45 out of 93 catchments show a time of emergence in the 21st century (Fig. 6a). An elevation dependence can be identified: times of emergence are earlier in higher-elevation catchments and later in lower-lying catchments. The high-Alpine catchments show particularly early times of emergence, with the end of the period 2017–2046 the earliest. The mean elevation of catchments with times of emergence earlier than 2065 is higher than 1500 m a.s.l., with one exception. Among the 48 catchments that do not show a time of emergence, 46 catchments have mean elevations lower than 1200 m a.s.l.

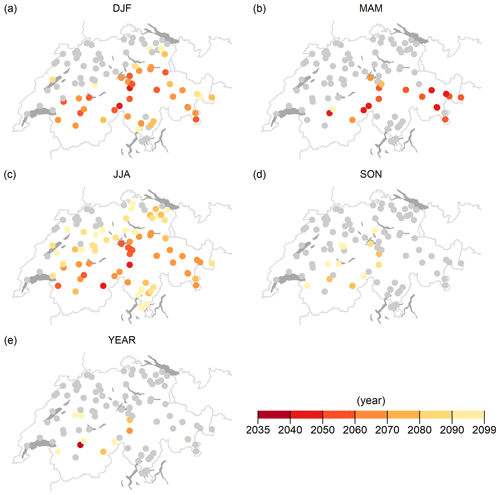
Figure 6 Time of emergence for winter (a), spring (b), summer (c), autumn (d), and year (e) when at least 66 % of the models agree on significant changes in the distribution of seasonal and annual means.

In summer, 73 catchments exhibit a time of emergence and again generally an earlier time of emergence for higher-elevation catchments (Fig. 6c). Catchments showing a time of emergence earlier than 2065 are all located in mountainous areas with mean elevations higher than 1500 m a.s.l., again with one exception. The earliest time of emergence in summer is found for the year 2043 (period 2014–2043). Catchments without time of emergence show a mean elevation lower than 1000 m a.s.l., with one exception.

In spring, only 20 catchments exhibit a time of emergence (Fig. 6b). Again, significant changes in the distribution are mainly found in the higher Alpine catchments (above 1500 m a.s.l.). Only 14 catchments out of 93 exhibit a time of emergence in autumn (Fig. 6d). In contrast to the other seasons, there is no clear elevation pattern distinguishable in autumn.

In all seasons, some of the catchments do not show a time of emergence, meaning that there is no statistically significant change in the distribution of the seasonal means. Clear patterns of significant changes in the distribution of seasonal means are mainly found in winter and summer.

For the annual means, 11 catchments reveal a time of emergence in the 21st century (Fig. 6e). Time of emergence in the annual mean is not restricted to high-Alpine catchments, but only two catchments below 1500 m a.s.l. show significant changes, which are after 2095.

Due to the definition of time of emergence as the last year of a moving window in which the Kolmogorov–Smirnov test is rejected for the first time, time of emergence is not necessarily given for all periods after the first detection. Figure 7 shows the temporal evolution of the time of emergence for the seasons under RCP8.5. Most of the catchments show persistent significant changes after the first detection of a time of emergence. However, few catchments do not reveal persistent significant changes after the first detection due to nonlinear changes in the runoff response to climate change. The problem of nonconstant rejections affects 17 catchments in winter, 3 catchments in spring, 25 catchments in summer, and 6 catchments in autumn. Most of these catchments show a persistent time of emergence for the rest of the century a few years after the first detection.

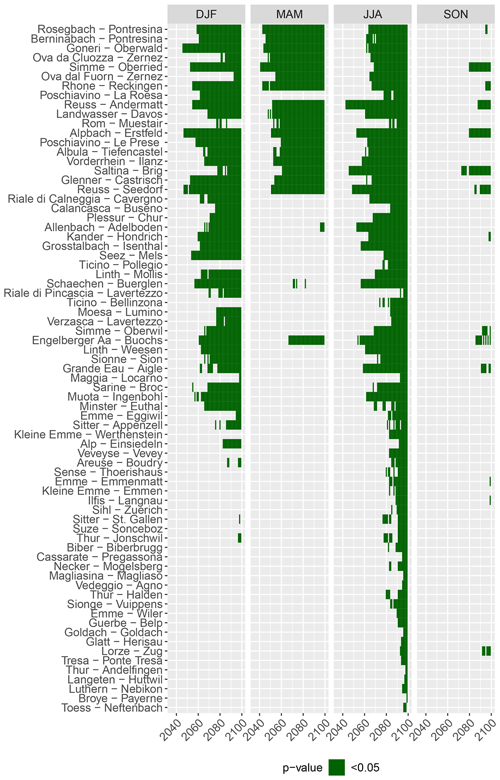
Figure 7 Temporal evolution of time of emergence for the seasons. Periods where the p value of the Kolmogorov–Smirnov test is lower than 0.05 are highlighted. Only catchments with at least one detection of time of emergence in one of the seasons are shown. Catchments are ordered by decreasing mean elevation of catchment.

'

4.4 Changes in seasonal means with increasing global mean temperatures

Changes in the multimodel median of seasonal and annual mean runoff for different levels of global warming are shown in Fig. 8 for warming targets +1.5, +2, and +3 ^∘C. Generally, the patterns of change with levels of global warming are similar to the patterns for the two emission scenarios. Both the range of change between the catchments and the climate model agreement increase with higher global warming levels.

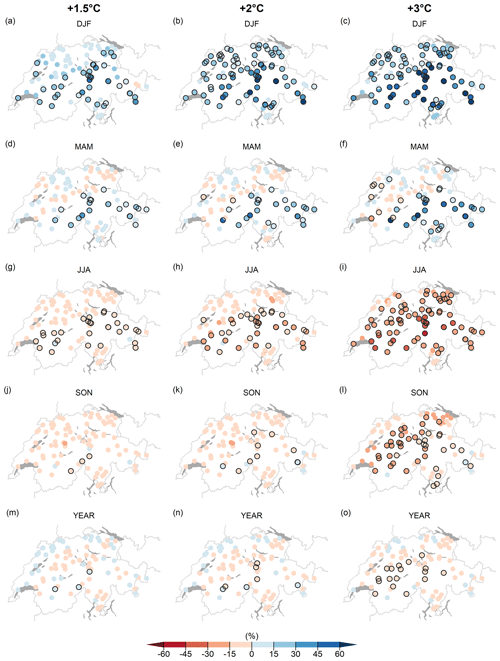
Figure 8 Multimodel median of seasonal and annual mean changes of runoff due to +1.5 ^∘C (a, d, g, j, m), +2 ^∘C (b, e, h, k, n), and +3^∘ C global warming for winter (a–c), spring (d–f), summer (g–i), autumn (j–l), and year (m–o). Black circles indicate catchments whose direction of change agrees across at least 90 % of the models.

In winter, the mean runoff change across all catchments is +17 % for a global warming of +1.5 ^∘C, +23 % for +2 ^∘C, and +35 % for +3 ^∘C (Fig. 8a–c). Stronger global warming increases not only the mean but also the range of change across the catchments. At +1.5 ^∘C global warming, the range across all catchments is between −1 % and +53 %, but at +3 ^∘C global warming, the range is +5 % to +127 %. Whereas two catchments show slightly negative changes at +1.5 ^∘C warming, all catchments show positive changes for higher warming levels. Moreover, the agreement across the climate models per catchment increases with increasing warming.

The mean change in spring runoff among all catchments is +4 % for +1.5 ^∘C global warming, +6 % for 2 ^∘C, and +10 % for 3 ^∘C (Fig. 8d–f). The changes in spring vary with elevation (see results in Sect. 4.1), with positive changes mainly for the higher-elevation catchments and negative changes for lower-lying catchments. Even though the average across all catchments changes little between warming levels, the range of change across the catchments and the model agreement per catchment increase with higher warming levels; thus, the regional elevation-dependent patterns become more pronounced.

In summer, the average among all catchments is −7 % for 1.5 ^∘C global warming, −13 % for 2 ^∘C, and −23 % for 3 ^∘C (Fig. 8g–j). Again, the ranges across catchments and the model agreement increase with higher warming levels. Compared to summer, the decrease in autumn runoff is smaller, with an average across all catchments of −9 % for 1.5 ^∘C global warming, −7 % for 2 ^∘C, and −13 % for 3 ^∘C. Most catchments, 89 out of 93, show a negative multimodel median for both 1.5 and 3 ^∘C warming levels. Although only three catchments show a model agreement of more than 90 % for 1.5 ^∘C global warming, 43 catchments show robust model agreement on the direction of change for 3 ^∘C global warming.

For annual mean runoff, the mean among all catchments is +0.2 % for 1.5 ^∘C, −0.9 % for 2 ^∘C, and −3.7 % for 3 ^∘C. Despite the slightly positive sign for 1.5 ^∘C warming, 53 catchments out of 93 show negative changes. This number increases to 66 catchments for 2 ^∘C and to 77 catchments for 3 ^∘C. Moreover, model agreement increases from 3 catchments with robust model agreement for 1.5 ^∘C to 17 catchments for 3 ^∘C.

5 Discussion

Winter runoff is projected to increase in Switzerland due to enhanced winter precipitation and increasing temperatures with climate change (CH2018, 2018). The higher temperatures result in more liquid precipitation and less solid precipitation in winter. This leads to less snow accumulation and thus to more direct runoff in winter. The relative changes in winter mean runoff in glaciated catchments are very high but still negligible as a contribution to the annual runoff volume. In nival and pluvial catchments, the contribution of winter runoff to the annual volume increases.

In spring, runoff in glaciated and nival catchments is projected to increase due to increased snowmelt, particularly in early spring. The combination of reduced snow accumulation in winter and earlier snowmelt shifts the peak in runoff regime to a month earlier. Lower-lying catchments show decreasing runoff in spring due to reduced snow accumulation in winter, which reduces the snow available for snowmelt.

The summer runoff in Switzerland generally decreases with climate change. The processes governing this decrease differ with location and elevation of the catchments. In lower-lying catchments, reduced summer precipitation and enhanced evapotranspiration result in decreasing runoff in Switzerland. In high-Alpine regions, where summer snowmelt and glacier melt dominate the runoff generation in the reference period, reduced snowpack and glacier retreat amplify the decrease in summer runoff. The large model spread in glaciated catchments in summer stems from the sensitivity of the runoff response to the glaciation of the catchment. In catchments where not all climate projections result in a complete disappearance of glaciers, the model spread increases, so future glacier retreat is a major source of uncertainty.

Autumn runoff is also projected to decrease due to increased evapotranspiration and slightly reduced precipitation in most catchments (CH2018, 2018). In a few of the eastern catchments, autumn precipitation slightly increases, but the effect of enhanced evapotranspiration and the reduced contribution from glacier melt and snowmelt are more dominant; autumn runoff also decreases in those catchments. Again, this decrease is amplified in catchments where glacier melt is important in early autumn under current climate conditions. However, the decrease in autumn runoff is most noticeable in early autumn, whereas in late autumn, at the end of October and November, the changes are less significant and can even change direction to positive values. This pattern is mainly found in very high Alpine catchments, where late autumn precipitation can fall more often as rain instead of snow and thus cannot be stored as snowpack. For the annual mean, a decrease in runoff was found in most catchments but with less robust signs among the climate models. This leads to the conclusion that, on the annual average, less water will be available in Swiss rivers. The shift in seasonality and thus a shift in the seasonal availability of water will impact many economic sectors. For example, increasing winter runoff may be beneficial for energy production, but decreasing summer runoff may lead to limitations in irrigation, particularly in lower-lying catchments where agricultural irrigation plays a crucial role.

In most catchments and seasons, the sign of change is the same for the high-emission scenario, RCP8.5, and the low-emission scenario, RCP2.6. Changes in seasonality or in the runoff regime are amplified by higher emissions and thus by increasing global mean temperatures. This amplification due to increased emissions and with intensified global warming shows the large benefits of mitigation. By mitigating climate change and following the RCP2.6 pathway, the magnitude of change can be reduced or even avoided, depending on the season and the runoff regime. However, some of the seasonal changes can no longer be avoided by mitigation actions, particularly in high-Alpine catchments with glacier influence. Responsible planners and policymakers need to adapt to this shift in the seasonal availability of water in our rivers.

Previous studies on climate change impacts on the runoff regime in Switzerland (e.g., Köplin et al., 2012, 2014; Horton et al., 2006) were driven by other emission scenarios, other and fewer climate model chains, different methods of postprocessing the climate model output, and/or different hydrological models and calibration. Despite these differences, the sign of change in those studies agrees in most seasons with the signs in this study. A pronounced dependence of runoff changes on elevation was also found by Köplin et al. (2012), with lower-lying catchments being less affected by climate change. The largest difference concerns the annual mean runoff. Köplin et al. (2012) found an increase (up to +50 %) in annual runoff for high-elevation catchments and no change for lower-lying catchments in the annual volume. In contrast, our study projects a decrease in the annual mean runoff not only for high-elevation catchments but also for most of the lower-lying catchments. However, not all catchments show a robust decrease among the climate model chains. This difference between our results and previous studies may arise from the use of the most recent generation of Swiss Climate Change Scenarios (CH2018), which project slightly different precipitation changes with less summer drying and wetter winters (CH2018, 2018) than the previous generation of scenarios, CH2011, which was used by Köplin et al. (2012). The different handling of glacier melt processes and the new projections of glacier extents (Zekollari et al., 2019) may also result in slightly different projections in glaciated catchments. The Hydro-CH2018-Runoff ensemble uses transient glacier projections that are updated every 5 years in the hydrological model. Köplin et al. (2012) used static glacier projections for 30 years. The largest uncertainties in glaciated catchments were also found by Addor et al. (2014) due to the different handling of glacier extents and resulting glacier melt. Furthermore, the difference in the input data (transient projections versus delta change projections with same baseline time series for the reference period) may add to the different signs.

Even though not all catchments show a time of emergence in the 21st century, significant changes in the distribution of seasonal means emerge particularly early in high-elevation catchments. This is due to the importance of snowmelt and glacier melt for Alpine runoff regimes. With climate change, the influence of snowmelt and glacier melt decreases due to higher temperatures and subsequent glacier retreat. Lower-lying catchments generally show a later time of emergence. This may arise from the large interannual variability in pluvial catchments in the reference period and in future periods. Köplin et al. (2014) also assessed a time of emergence for Swiss catchments but only based on significant changes for two scenario periods and 10 climate models. Since their climate models were postprocessed with a delta change approach, only the natural variability of the reference period is reflected in their simulations. Despite these differences in methodology and data, they also found earlier time of emergence in winter and summer for high-elevation catchments. Although the definition of time of emergence applied here is commonly used in other climate change studies (e.g., Mahlstein et al., 2011), this definition also has disadvantages. For example, if the rejection of the null hypothesis is unstable in its temporal evolution, the time of emergence may be determined too early. This has been shown for some of the catchments in the present study. However, in most catchments a persistent detection of time of emergence (p value < 0.05) in the distribution of the seasonal means was found shortly after the first detection of a time of emergence.

The findings of this study concern runoff regime changes for Switzerland. The pronounced changes in high-elevation catchments highlight the important influence of temperature changes on snowmelt- and glacier-melt-driven catchments; thus, they also indicate that similar runoff responses may be found in other snow- and glacier-dominated regions. The results for lower-lying catchments, which are mainly driven by evapotranspiration and precipitation changes, may not be directly transferable to other regions. The runoff response in such catchments depends on local precipitation patterns and their changes under climate change.

Various sources of uncertainty affect our results. A detailed discussion of the uncertainties for the simulations is provided in Muelchi et al. (2021). Uncertainties arise from all the steps in the modeling chain: the scenarios of greenhouse gas concentrations, the climate models and their boundary and initial conditions, the postprocessing method (Gutiérrez et al., 2019), the hydrological model (Addor et al., 2014) and its calibration, and the underlying glacier projections; all these need to be considered in adaptation planning (e.g., Wilby and Dessai, 2010). Working with three emission scenarios and an ensemble of climate models partly addresses some of the uncertainty issues. Muelchi (2021) compared the results for three catchments to simulations from three different versions of hydrological models, and the key findings are robust among the models and the catchments.

6 Conclusions

Changes in runoff regimes and their time of emergence were assessed with the new hydrological scenarios for 93 catchments in Switzerland. This study is based on the most recent generation of climate change scenarios, postprocessed with quantile mapping. Compared to previous studies on runoff regime changes, the results show similar signs of change for most seasons. The largest differences were found for the high-elevation catchments, which is likely due to the transient characteristic of the simulations and the implementation of transient glacier projections driven by the same climate model chains.

In general, winter runoff is projected to increase and summer runoff to decrease in Switzerland. The sign of change is robust across catchments, but the magnitude of change is more pronounced for high-elevation catchments. Particularly in summer, when snowmelt and glacier melt play an important role in runoff generation, glaciated catchments will face a strong decrease in runoff due to the retreating glaciers. In rainfall-dominated catchments, the changes are also often robust but at a lower magnitude. Whereas the higher-elevation catchments show increasing spring runoff due to earlier snowmelt, the pluvial catchments in the lowlands will face decreasing spring runoff. A decrease in runoff is also found for autumn and annual mean runoff in most catchments. These seasonal patterns amplify with global warming and with higher-emission scenarios. Furthermore, the model agreement among the climate models increases with the strength of the emission scenario and the distance in time. A time of emergence of seasonal mean runoff was mainly found in summer and winter and for only a few catchments in spring and autumn. Early times of emergence, before 2060 in winter and before 2065 in summer, were found for catchments above 1500 m a.s.l. Significant changes in catchments below 1500 m a.s.l. emerge later in the century. However, not all catchments show a time of emergence in all seasons.

The amplification of changes by enhanced global warming highlights the importance of climate change mitigation. By mitigating climate change and following the RCP2.6 pathway to keep global warming below 2 ^∘C, the magnitude of change can be reduced substantially. The large decrease in summer runoff in glaciated catchments can be strongly dampened but not avoided entirely, because glacier retreat is projected even for the low-emission scenario. The present study can help to support adaptation planning in various sectors by presenting detailed information on changes in mean runoff.